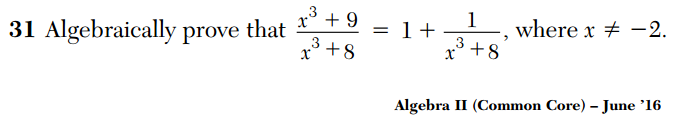Teaching

In my ongoing analysis of New York State’s math Regents exams, the most discouraging issues I encounter are the mathematical errors.  Consider this two-point problem from the June 2016 Common Core Algebra 2 exam.

There are no issues with this simple algebraic identity, but there is a serious issue with how this problem was graded.

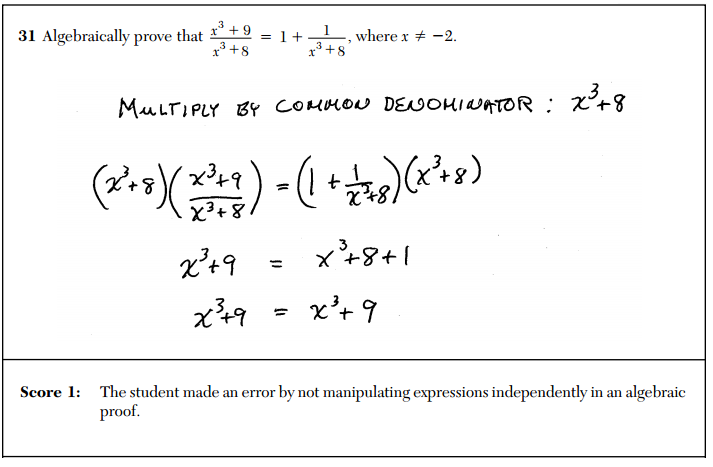
With each exam a set of model responses are published by the state.  These serve as exemplar student work and inform graders of what correct and incorrect responses look like.  Here is a model response for this problem.

Sadly, according to the official scoring guidelines, this perfectly valid and 100% correct response earns only one point out of two.

The purported reason for the penalty is that “The student made an error by not manipulating expressions independently in an algebraic proof”.  It’s unclear what, if anything,  this means, but there is no requirement that expressions be manipulated independently in an algebraic proof.  This is an artificial criticism.

I suspect the complaint has to do with multiplying both sides of the equation by some quantity.  I have occasionally heard teachers argue that, when proving an identity, you can’t multiply both sides of an equation by the same thing.  Their reasons vary, but the most common explanation is that in doing so you are assuming the sides are equal, which is what you are trying to prove.

This is faulty mathematics.  For the most part, there is no issue with multiplying both sides of a purported identity by the same quantity:  if the original equation is true, the new equation will be true, and if the original equation is false, the new equation will be false.  In general, the equations are logically equivalent, that is, true and false under exactly the same circumstances.

For example, consider the true equation 4 = 4 and the false equation 4 = 5.  Notice that

4 = 4   and   3*4 = 3*4

are both true, and

4 = 5   and   3*4 = 3*5

are both false.  Multiplying both sides by 3 does not change the truth value of either equation.

Now, I said for the most part because there is one particular situation in which multiplying both sides of an equation by the the same quantity can be problematic.  Multiplying both sides of an equation by zero can turn a false equation into a true equation.  For example

3 = 2

is clearly false, but

 0*3 = 0*2

is true.

So, the full mathematical story is that the statements a = b and ka = kb are logically equivalent if .  That is to say, multiplying both sides of an equation by the same quantity will preserve its truth value if you aren’t multiplying by zero.

Let’s return to the model response in question.  We have

Here, both sides of the original equation have been multiplied by .  As long as , these two equations are logically equivalent, and so proving that the latter equation is an identity is equivalent to proving that the original equation is an identity.

Could  equal 0?  Generally speaking, yes.  But conveniently, the exam authors went to the trouble to tell us that , which means .  Therefore, the mathematics of the model response is perfectly valid.  It should earn full credit.

The error in these official grading instructions demonstrates a serious lack of mathematical understanding on the part of those who produce these exams.  Errors like this, and this, and this, should give pause to those who automatically assume that exams like these are valid measurements of mathematical knowledge and ability.

And there are serious and real consequences here.  Not only are student losing points for perfectly valid mathematical work, official state documents are demonstrating incorrect mathematics to classroom teachers.  Without correction, this erroneous mathematics will likely be passed along to students.

While there can be legitimate disagreement and debate about the extent and use of testing in education, I think we can all agree that the tests themselves should not undermine the teaching and learning of content.  And that’s exactly what errors like this do.

Related Posts

• Regents Recaps
• Yes, You Can Work on Both Sides of an Identity
• The Worst Regents Question of All Time
• More Trouble With Functions
• Another Embarrassingly Bad Math Exam Question

“Simplest form” is a dangerous phrase in math class.  Whether a form of an expression is simple or not depends on context.  For example, while and are representations of the same number, the first fraction is likely to be seen as simpler than the second.  But if the goal were, say, to determine if the number was greater than , then the expression on the right might be considered simpler.

Despite the wide and varied uses of the phrase “simplest form”, I have never heard it used in the context of complex numbers.  So I was surprised by this Common Core Algebra 2 Regents exam question.

I don’t know what the author of this question means here by “simplest form”.  I asked around, and someone suggested that the natural interpretation of “simplest form” here is a + bi.  That seems reasonable, but since none of the answers are in a + bi form, the author of this question could not have meant that.  [It is also worth noting the implicit assumption here that y is a real number, an issue that has come up before on these exams].

What’s most bothersome about this imprecise use of language is that it is completely irrelevant to this question.  Whatever “simplest form” means here, it is of no consequence:  there is no answer choice which is otherwise correct but in some improper form.

The question should simply ask which expression is equivalent to the given expression.  The use of “simplest form” here not only obfuscates the mathematics of the problem, but models imprecise use of mathematical terminology.  We should expect our high stakes exams to do better.

Related Posts

• Regents Recaps
• Regents Recap — June, 2012: Erroneous Questions
• Regents Recap — June, 2013: Encouraging Bad Habits