Regents Recap — August 2017: Yes, You Can Work on Both Sides of an Identity
In a controversial post last year, I argued that it’s perfectly acceptable to work on both sides of an equation in proving an algebraic identity. While it’s common to tell students “You can’t cross the equal sign” in this situation, doing so is mathematically legitimate as long as the new equation is true under exactly the same circumstances as the original.
For example, when proving an algebraic identity, multiplying both sides of an equation by 2 is permissible, because x = y and 2x = 2y are true under exactly the same conditions on x and y. Squaring both sides of an equation however, is not, since
can be true under conditions that make x = y false, say, when x = 2 and y = -2.
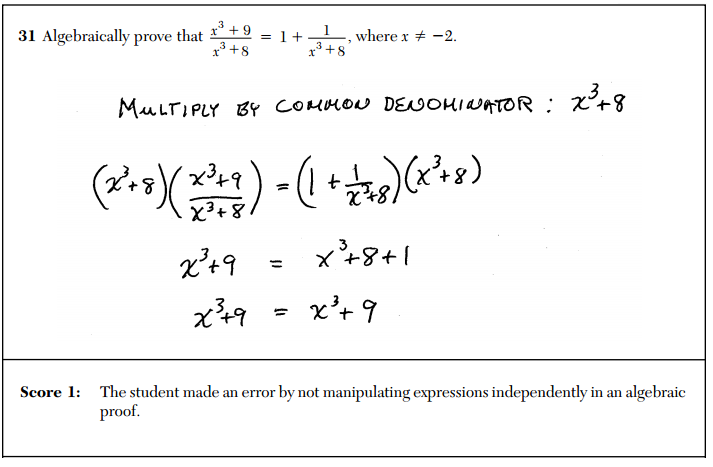
The post in question, “Algebra is Hard”, was a response to a June 2016 Regents scoring guide that deducted a point from a student who, in proving an algebraic identity, multiplied both sides of the equation by a non-zero quantity. The student was penalized for “not manipulating expressions independently in an algebraic proof“, a vague and meaningless criticism.
“Algebra is Hard” received quite a bit of attention, and while many agreed with me, I was genuinely surprised at how many readers disagreed. Which was terrific! Of course my argument makes perfect sense to me, but it was great to have so many constructive conversations with teachers and mathematicians who saw things differently.
But my argument recently received support from the most unlikely of sources: another Regents exam.
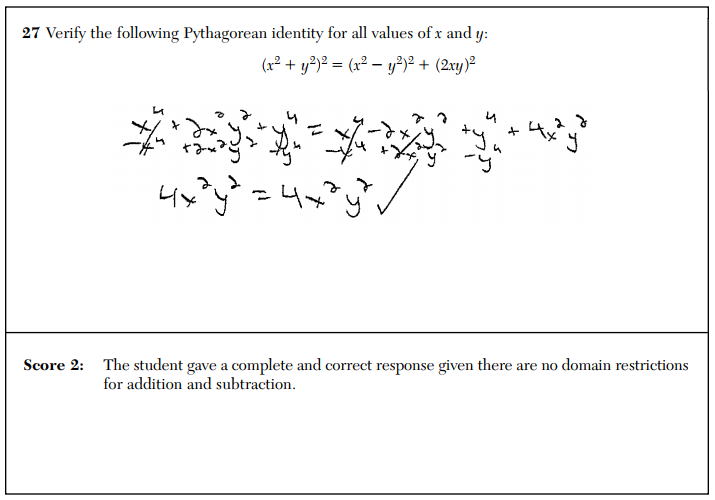
Take a look at this exemplar full-credit student response to an algebraic identity on the August 2017 Algebra 2 exam.
Notice that the student works on both sides of the equation and subtracts the same quantity from both sides. Even though the student did not manipulate expressions independently in an algebraic proof, full credit was awarded.
The note here about domain restrictions is an amusing touch, given that it was the explicit domain restriction in the problem from 2016 that ensured the student wasn’t doing something impermissible (namely, multiplying both sides of an equation by 0).
So in 2016 this work gets half credit, and in 2017 this work gets full credit.
While it’s nice to see mathematically valid work finally receiving full credit on this type of problem, it’s no consolation to the many students who lost points for doing the same thing the year before. What’s especially frustrating is that, as usual, those responsible for creating these exams will admit no error nor accept any responsibility for it.
Be sure to read “Algebra is Hard” (and some of the 40+ comments!) for more of the backstory on this problem.
Related Posts
2 Comments
Danny · October 23, 2017 at 8:51 pm
I see this slightly different, I don’t see a change in the scoring. For the 2017 question it specifically states that there are no domain restrictions for addition and subtraction. While, there are restrictions for multiplication. I wonder if in the 2016 question the student would have received full marks if he had stated that x³+8 ≠ 0 for x≠-2.
If they are looking for rigorous proofs, I can see where that step would be required.
MrHonner · October 24, 2017 at 8:21 am
This is absolutely a change in scoring. As pointed out, the rationale behind the deduction in 2016 was a failure to “manipulate expressions independently”. The student in 2017 also failed to “manipulate expressions independently”, but was awarded full credit.
The question of rigor is always dependent on context. And the question of what they (i.e., those responsible for these exams) are looking for is a matter of what is communicated in the test guidelines, scoring rubrics, and model response sets. And what is being communicated here is incorrect and inconsistent.