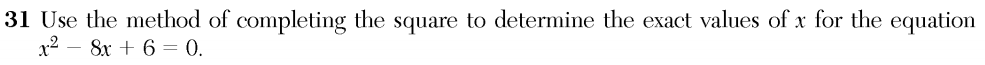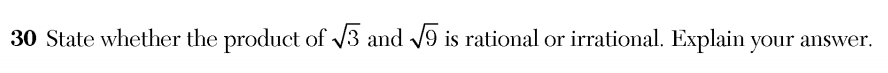In a past life I worked in office buildings. One of the reasons I quit that life was the inescapable perpetuity of it all. There was no end, no beginning. Just work.
The well-defined beginnings and ends of the school year are important to me. Anticipation creates excitement, closure creates opportunities to reflect. Working in perpetuity made me realize how important this cycle was in my life.
I always have my students write end-of-year reflections, in part to bring their attention to this cycle, but also because there are questions I don’t know the answers to. And I want to know. Although I could have guessed many of those answers.
What worked for you? Breakout rooms, because I got to learn from others and build relationships with my classmates.
What didn’t work for you? Breakout rooms, because they were awkward and no one talked.
What did you learn about yourself? I learned that I need social interaction more than I thought.
How do feel about next year? I’m nervous that I won’t recognize any of my classmates because I don’t know how tall anyone is. (Ok, I never would have guessed that.)
What would have improved your experience this year? In-person classes. (No surprise there.)
My own end-of-year reflection begins with “I made it”. I think this is where many teachers would start their self-evaluations. But as I look deeper, I realize how far I’ve come, how much I’ve learned, and how many new skills I’ve developed. I now feel comfortable teaching a live remote class. I have a sense of how to structure asynchronous learning, and knowledge of tools that can make it meaningful. I can build relationships with students in a virtual environment. I can run a workshop for teachers in zoom. I can facilitate teacher teams remotely.
I couldn’t say any of these things twelve months ago. It feels good to be able to say them now at the end, and at the start of a new beginning.
Related Posts