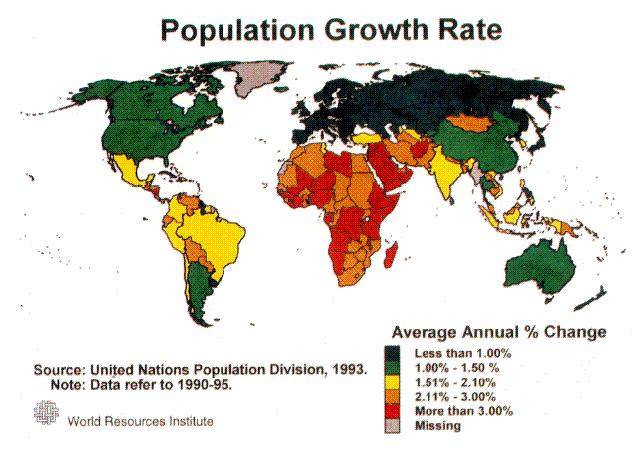
Here’s an introduction to the one-cut challenge using triangles, from my Fun with Folding series, suitable for students of all ages (including teachers!). This is a rich, compelling problem that touches on a lot of sophisticated ideas in geometry, but is simple enough to start playing around with right away.
The one-cut challenge is as follows: given a shape made up of connected straight line segments (i.e. a polygonal chain), can you produce the shape as a cut-out using only straight folds and a single straight cut?
A good place to start is with an equilateral triangle. This is a fairly easy problem to solve, given the inherent symmetry in the figure. Fold across any line of symmetry to produce a new figure that looks like two line segments meeting at an angle. Fold those together along their vertex, and cut!
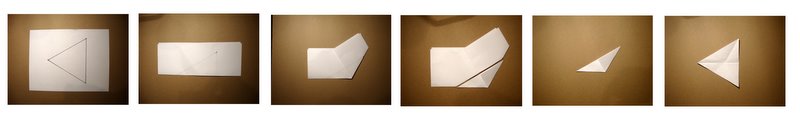
The next step is trying this with an isosceles triangle, whose single line of symmetry still allows this approach to work.

Now the kicker: try it out on a scalene triangle! No more lines of symmetry, and all of the sudden this is a pretty challenging problem!
Happy folding!
Have more Fun With Folding!
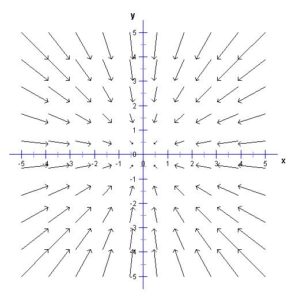 This is a great tool for plotting vector fields:
This is a great tool for plotting vector fields: