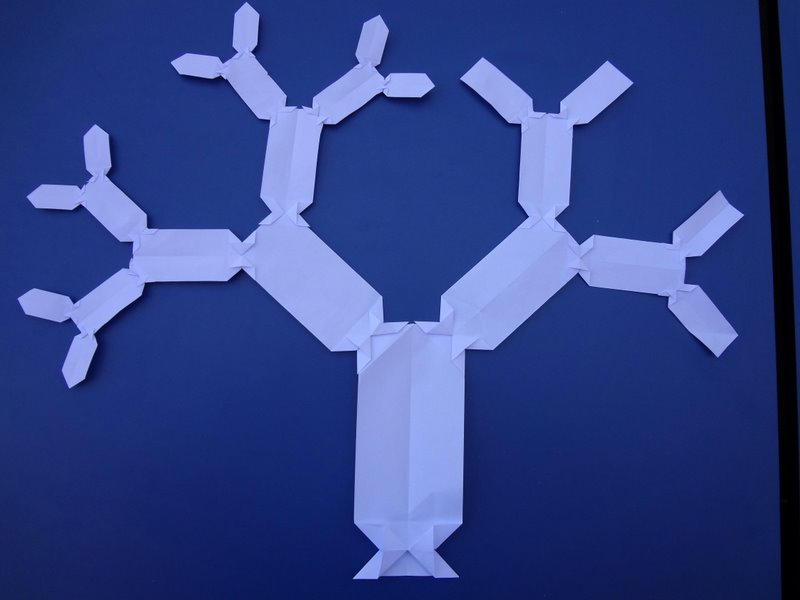
Here’s another great idea I got from the Bridges Math and Art conference: fractal origami. Check out this folded version of Pythagoras’s Tree.
Apparently, the dimensions of A1 paper are such that if you cut the rectangle in half, parallel to the shorter side, the result will be two rectangles that are roughly similar to the original. This self-similarity allows you to repeat the cutting and folding process, producing smaller and smaller branches.
Have more Fun With Folding!
Studying vector calculus tends to make you see space curves everywhere you go. Here’s a conical helix (or a helical cone?).
A good way to understand the behavior of curves in space is to understand how their projections behave. The sun does a nice job of showing us one such projection of this space curve.
This suggests a common mathematical practice: trading a hard problem for an easier one. Space curves can be difficult to analyze, but their projections are more easily understood. And by understanding its projections, you can develop knowledge of the space curve itself.
Of course, it’s important to understand what information you lose through the projection, as well!
In honor of Pi Day, here’s a wonderful example of student creativity: a necklace that encodes the first 80 or so digits of pi in beads!
Starting with the pendant as 3, the beads and string clockwise, in a circle, according to the following mapping:
Thus, you can read off 3.14159265358, and so on. A truly thoughtful, creative, and inspired work!
Related Posts
This is a sample of the problems from the book “200 Puzzling Physics Problems” published by Cambridge University Press:
http://catdir.loc.gov/catdir/sam ples/cam034/00053005.pdf
This above resource contains 89 of the problems, but no solutions.
The problems here are simple to state, but seem to get at profound mathematical and physical ideas. For example, if the Earth and Sun were scaled down as to be 1 meter apart on average, how long would a year be?
I can see a lot of productive student struggle coming from this book!
