Mr Honner

The Self-Replicating Printer

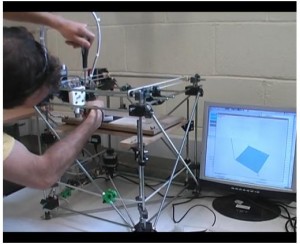
After reading a post about 3-D printers, I found out about this assemble-it-yourself 3-D Printer!

Not only is the RepRap cheap to build (people claim it can be done for around $400), but all the software and hardware designs are open-source and free.

But it doesn’t stop there. If you already have a RepRap, you can use it to build some of the parts you to build another RepRap! In other words, this is a self-replicating machine! Well, partially at least.

I’m sure there are plenty of people out there with the skills to build one of these themselves. I’m not sure I’m one of them–after all, building a table that was almost level was a huge success for me. But this is such a cool idea it might just be worth a shot!

By patrick honner, 14 years9 years ago

Challenge Economics

Are Stock Prices Random?

A lot of people spend a lot of time trying to understand stock prices: Are they predictable? Are they random? Can you make money by identifying trends? Can you beat the market and make a fortune?

A prevailing theory is that stock prices are essentially random walks; that is, no more predictable than a coin flip. The amount a price goes up or down at any given moment might follow some pattern (small movement is more likely than large movement, for example), but whether that movement is up or down is basically random. Now, what random means to mathematicians can get kind of complicated, but that’s another story.

I thought it might be interesting to compare actual stock prices to a randomly-generated trend line. After playing around with a spreadsheet and experimenting with different parameters, I produced the following two graphs:

One of these graphs represents 200 days of prices of the Dow Jones Industrial average; the other represents a quantity that moves up or down randomly, by some random amount. Figuring out how to get a good-looking random graph took some time, and is an interesting challenge in and of itself.

So, can you tell which is the Dow and which is a coin toss? More importantly, how much would you be willing to bet on it?

Related Posts

Are Stock Prices Random? Part II

By patrick honner, 14 years7 years ago

Challenge Geometry Student Work Teaching

An Impossible Construction

I enjoy offering impossible problems to students as extra credit, although I usually don’t tell them the problems are impossible. Such tasks usually engage them, confuse them, and make them suspicious of me. It’s a win-win-win.

While discussing some three-dimensional geometry, I offered extra credit to anyone who could build a model of a Klein bottle. The Klein bottle is a hard-to-imagine surface that has neither an inside nor an outside. It’s like a tube where one end meets the other and makes a seal, but somehow got turned inside out in the process. If you are familiar with the Mobius strip, the Klein bottle is basically a higher-dimensional Mobius strip.

One reason that the Klein bottle is hard to visualize is that it can’t be observed in three dimensions: it needs a fourth dimension in order to see it turn itself inside-out. This is analogous to the standard construction of the Mobius strip: we take a long strip of paper, give one end a half-twist, and tape the ends together. We think of the paper itself as being 2-dimensional, but we need that third dimension to twist through.

So, I was pretty impressed with the student who made this.

Not bad at all, for someone who is dimensionally challenged. Here’s a nice representation for comparison, although it’s still a cheat. The Klein bottle doesn’t really intersect itself.

A nice example of impossibly creative student work!

By patrick honner, 14 years9 years ago

Application Technology

Plotting the Sun’s Path

Here’s a cool article from the Wolfram Alpha Blog about using WolframAlpha to plot the sun’s path, as seen from various locations:

http://blog.wolframalpha.com/2010/09/22/following-the-suns-unique-path/

You can also alter the date, which means you can look at historical data or projected future data.

Unfortunately, when I tried the command “sunpath north pole” (employing the classic mathematical strategy consider extreme cases), WolframAlpha gave me the sun path for North Pole, Alaska, a very oddly named place as it is nowhere near the actual North Pole.

By patrick honner, 14 years9 years ago

Appreciation Geometry

Some Strange Circles

I was trying to construct a simple, introductory intersection problem for the first day of Calculus class, so I started with a well-behaved circle:

This is a circle centered at (3,2) with radius 5. I picked two points on the circle, (0,6) and (7,-1), found the equation of the line between them, and put together my system of equations:

So I had successfully reverse-engineered my circle-and-line intersection problem with two nice solutions: (0,6) and (7,-1).

Unfortunately, I made a typo on the handout. At the end of the left side of the circle equation I wrote ” + 12″ instead of ” – 12″.

So all my work was for naught. Or so I thought. Turns out, at least two amazing things happen:

First, the new circle still ends up having a nice radius, namely 1. What’s even more amazing is that the new circle also ends up having two nice intersections with the given line, (3,3) and (4,2)!

I wish my intentional work always turned out as well as this mistake!

By patrick honner, 14 years9 years ago

Follow Mr Honner