Mr Honner

Curvefitting With Geogebra

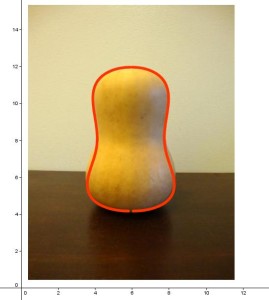
Inspired by some of my own forays into curvefitting with Geogebra (the squash at the right, or my Sine Waves on the Beach), I’ve created a student project built around the idea.

Finishing up a unit on trigonometry with graphs of trigonometric functions, it occurred to me that I have never really been comfortable teaching transformations. I think part of the reason is that it’s hard to get your hands dirty, play around, and develop intuition with this topic. This is where Geogebra comes in!

The project essentially works like this:

1) Students find an image, preferably one they capture themselves

2) Students paste the image into Geogebra

3) Students graph a relevant trigonometric function and play around with the various parameters (like period, amplitude, phase shift) until the curve fits the image

4) Students can use domain restrictions, and some of Geogebra’s aesthetic features, to polish everything up.

The first run of this project has produced some great results! You can see some sample student work here, and more on my Facebook page.

Resources Technology

Library of Visual Manipulatives

This is another terrific resource: the Library of Visual Manipulatives from Utah State University.

http://nlvm.usu.edu/

There are one hundred or so simple, interactive programs available spanning Algebra, Geometry, Numbers and Operations, Measurement, and Data. And everything is sorted by appropriate age level. There are certainly a lot of interesting activities to navigate through here.

I’d say this is NSF money well-spent.

By patrick honner, 14 years8 years ago

Geometry Resources Technology

Toothpick Sequences

This is a cool applet that allows you to explore various fractal “toothpick sequences”:

http://www2.research.att.com/~david/oeis/toothpick.html

A number of options allow the user to look at variants of the object, zoom in, change iteration parameters, and change the underlying sequence. Click the Introduction button for a short overview.

Not sure what ATT Research plans to do with this, but it’s fun to play around with!

By patrick honner, 14 years8 years ago

Geometry

CDs and Cavalieri’s Principle

I finally got around to shedding my library of CD cases (I know, I’m quite behind), but it got me thinking about Cavalieri’s Principle.

Cavalieri’s Principle essentially states that if two prisms have the property that all corresponding cross sections have the same area, then those prisms have the same volume.

For instance, here we have two stacks of CD cases. Every cross-section of each “prism” here is a single CD case. Since corresponding cross-sections always have equal area, Cavalieri’s Principle tells us that these prisms have equal volume, even though one of the stacks is oblique.

That the stacks have equal volume is made clearer with a simple transformation of the stack on the right.

Here’s another demonstration of Cavalieri’s Principle using the CDs themselves.

Challenge NYT Sports

Math Quiz: NYT Learning Network

Through Math for America, I am part of an on-going collaboration with the New York Times Learning Network. My latest contribution, a Test Yourself quiz-question, can be found here:

http://learning.blogs.nytimes.com/2011/04/4/test-yourself-math-april-4-2011/

This question is based on the financial troubles facing the New York Mets, and their contributions to baseball’s revenue sharing pool.

By patrick honner, 14 years8 years ago

Resources Teaching Technology

Curvefitting With Geogebra

Resources Technology

Library of Visual Manipulatives

Geometry Resources Technology

Toothpick Sequences

Geometry

CDs and Cavalieri’s Principle

Challenge NYT Sports

Math Quiz: NYT Learning Network

Follow Mr Honner