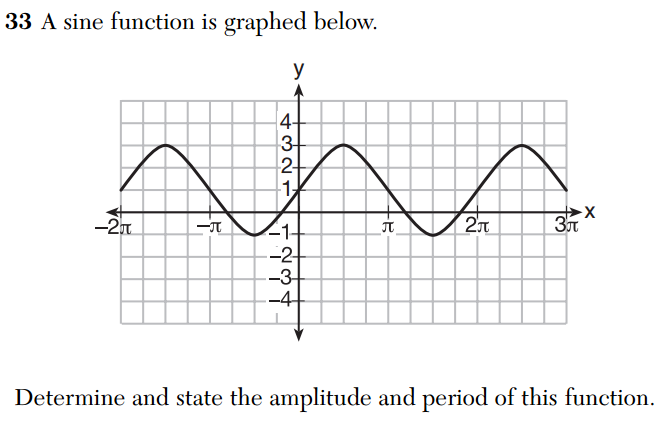
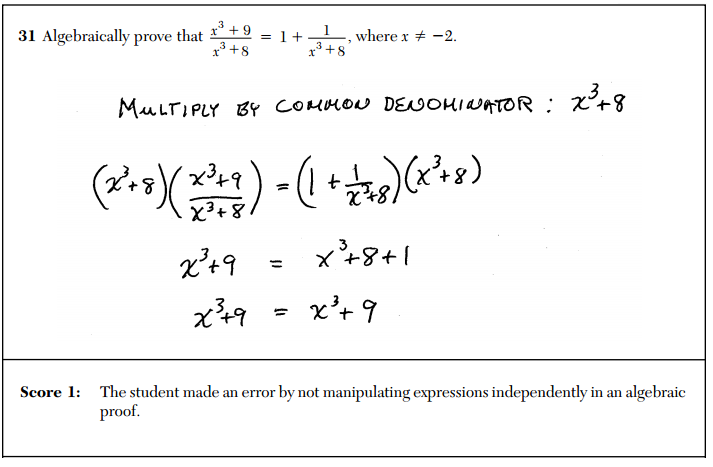
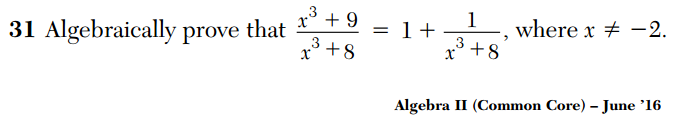Testing

Teaching Testing

Regents Recap — June, 2017: More Mathematical Misunderstanding

Far too often questions on New York State math exams demonstrate a disturbing lack of content knowledge.

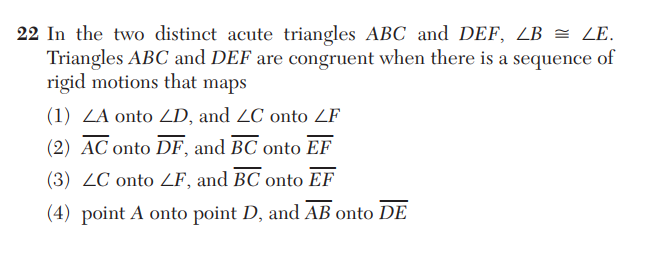
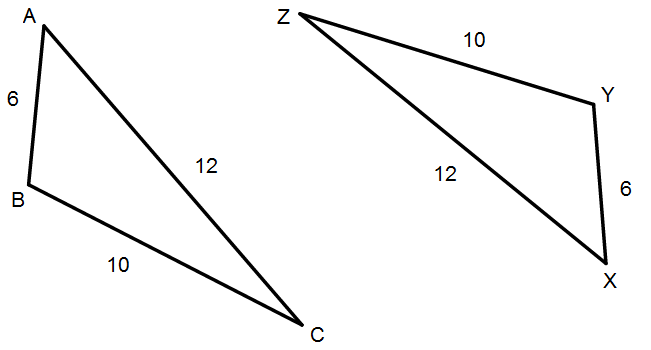
Consider this multiple choice item from the June, 2017 Common Core Geometry exam.

Instead of testing a student’s understanding of triangle congruence, this question exposes a serious lack of mathematical understanding among the exam creators.

A superficial reading of the problem suggests that (3) is the correct answer. In (1), the two triangles share only three pairs of congruent angles; in (2), two sides and a non-included angle are congruent in each triangle; and in (4), the triangles share only one pair of congruent sides and one pair of congruent angles. None of these scenarios (AAA, SSA, SA) seems sufficient to guarantee that the triangles are congruent. And in (3), the triangles have one pair of congruent sides and two pairs of congruent angles; this (ASA or SAA) is sufficient to conclude the triangles are congruent, so (3) is apparently the correct answer.

But closer inspection shows that, in fact, (1) and (2) are correct as well.

Consider choice (1). While it’s not exactly clear what it means to map angle A onto angle D, it must require that point A gets mapped to point D. Similarly, point C must be mapped to point F. If a rigid motion maps A to D and C to F, then segment AC must be congruent to segment DF. We now have one pair of congruent sides and three pairs of congruent angles: the triangles are congruent (by ASA or SAA), and choice (1) is a correct answer.

In (2), we are given that segment AC is mapped onto segment DF. This means that point A gets mapped to point D and point C gets mapped to point F. And since segment BC is mapped onto segment EF, we know that B is mapped onto E. Therefore, the vertices of triangle ABC are mapped via rigid motion onto the vertices of triangle DEF. This is sufficient to conclude that the triangles are congruent, and choice (2) is also a correct answer. (It’s also worth noting that, since the triangles are given as acute, SSA is actually sufficient to guarantee that the triangles are congruent. This mathematical error turned up in a separate question on this exam.)

As it stands, the only option that is not a correct answer to this question is (4).

Within a few days, the NYS Education Department issued a directive to count all answers to this question as correct. As is typical, no admission of an error was made: the problem was blamed on discrepancies in wording. Of course, there are no discrepancies in wording here: this problem as written, reviewed, edited, and ultimately published is simply mathematically incorrect. Its existence demonstrates a fundamental misunderstanding of the underlying concepts.

This isn’t the first time an erroneous question has made it onto one of these high-stakes Regents exams. In fact, there were at least three mathematically invalid questions on this exam alone! Over the past five years I’ve documented many others, and each time it happens, it raises serious questions: Questions about the validity of these exams, how they are experienced by students, how they are scored, and the lack of accountability for those in charge.

Related Posts

By MrHonner, 7 years7 years ago

Teaching Technology Testing Uncategorized

Regents Recap — June 2016: Still Not a Trig Function

I don’t know exactly why, but fake graphs on Regents exams really offend me. Take a look at this “sine” curve from the June, 2016 Algebra 2 Trig exam.

Looking at this graph makes me uneasy. It’s just so … pointy. Here’s an actual sine graph, courtesy of Desmos.

Now this fake sine curve isn’t nearly as bad as these two half-ellipses put together, but I just don’t understand why we can’t have nice graphs on these exams. It only took me a few minutes to put this together in Desmos. Let’s invest a little time in mathematical fidelity.

Related Posts

By MrHonner, 8 years8 years ago

Teaching Testing

Regents Recap — June 2016: Algebra is Hard

In my ongoing analysis of New York State’s math Regents exams, the most discouraging issues I encounter are the mathematical errors. Consider this two-point problem from the June 2016 Common Core Algebra 2 exam.

There are no issues with this simple algebraic identity, but there is a serious issue with how this problem was graded.

With each exam a set of model responses are published by the state. These serve as exemplar student work and inform graders of what correct and incorrect responses look like. Here is a model response for this problem.

Sadly, according to the official scoring guidelines, this perfectly valid and 100% correct response earns only one point out of two.

The purported reason for the penalty is that “The student made an error by not manipulating expressions independently in an algebraic proof”. It’s unclear what, if anything, this means, but there is no requirement that expressions be manipulated independently in an algebraic proof. This is an artificial criticism.

I suspect the complaint has to do with multiplying both sides of the equation by some quantity. I have occasionally heard teachers argue that, when proving an identity, you can’t multiply both sides of an equation by the same thing. Their reasons vary, but the most common explanation is that in doing so you are assuming the sides are equal, which is what you are trying to prove.

This is faulty mathematics. For the most part, there is no issue with multiplying both sides of a purported identity by the same quantity: if the original equation is true, the new equation will be true, and if the original equation is false, the new equation will be false. In general, the equations are logically equivalent, that is, true and false under exactly the same circumstances.

For example, consider the true equation 4 = 4 and the false equation 4 = 5. Notice that

4 = 4 and 3*4 = 3*4

are both true, and

4 = 5 and 3*4 = 3*5

are both false. Multiplying both sides by 3 does not change the truth value of either equation.

Now, I said for the most part because there is one particular situation in which multiplying both sides of an equation by the the same quantity can be problematic. Multiplying both sides of an equation by zero can turn a false equation into a true equation. For example

3 = 2

is clearly false, but

0*3 = 0*2

is true.

So, the full mathematical story is that the statements a = b and ka = kb are logically equivalent if $k \neq 0$ . That is to say, multiplying both sides of an equation by the same quantity will preserve its truth value if you aren’t multiplying by zero.

Let’s return to the model response in question. We have

$\frac{x^3 + 9}{x^3 + 8 } = 1 + \frac{1}{x^3+8}$

$(x^3 + 8)(\frac{x^3 + 9}{x^3 + 8 }) = (x^3 + 8)(1 + \frac{1}{x^3+8})$

Here, both sides of the original equation have been multiplied by $(x^3 + 8)$ . As long as $x^3 + 8 \neq 0$ , these two equations are logically equivalent, and so proving that the latter equation is an identity is equivalent to proving that the original equation is an identity.

Could $x^3 + 8$ equal 0? Generally speaking, yes. But conveniently, the exam authors went to the trouble to tell us that $x \neq -2$ , which means $x^3 + 8 \neq 0$ . Therefore, the mathematics of the model response is perfectly valid. It should earn full credit.

The error in these official grading instructions demonstrates a serious lack of mathematical understanding on the part of those who produce these exams. Errors like this, and this, and this, should give pause to those who automatically assume that exams like these are valid measurements of mathematical knowledge and ability.

And there are serious and real consequences here. Not only are student losing points for perfectly valid mathematical work, official state documents are demonstrating incorrect mathematics to classroom teachers. Without correction, this erroneous mathematics will likely be passed along to students.

While there can be legitimate disagreement and debate about the extent and use of testing in education, I think we can all agree that the tests themselves should not undermine the teaching and learning of content. And that’s exactly what errors like this do.

Related Posts

By MrHonner, 8 years3 years ago

Regents Recap — June 2016: How Much Should This Be Worth?

The following problem appeared on the June 2016 Common Core Algebra 2 Regents exam.

This is a straightforward and reasonable problem. What’s unreasonable is that it is only worth two points.

The student here is asked to construct a representation of a mathematical object with six specific properties: it must be a cosine curve; it must be a single cycle; it must have amplitude 3; it must have period $\pi / 2$ ; it must have midline y = -1; and it must pass through the point (0,2).

That seems like a lot to ask for in a two-point problem, but the real trouble comes from the grading guidelines.

According to the official scoring rubric, a response earns one point if “One graphing error is made”. Failure to satisfy any one of the six conditions would constitute a graphing error. So a graph that satisfied five of the six required properties would earn one point out of two. That means a response that is 83% correct earns 50% credit.

It gets worse. According to the general Regents scoring guidelines, a combination of two graphing errors on a single problem results in a two-point deduction. That means a graph with four of the six required properties, and thus two graphing errors, will earn zero points on this problem. A response that is 66% correct earns 0% credit!

The decision to make this six-component problem worth two points creates a situation where students are unfairly and inconsistently evaluated. It makes me wonder if those in charge of these exams actually considered the scoring consequences of their decision, especially since there are two obvious and simple fixes: reduce the requirements of the problem, or increase its point value.

This is another example of how tests that are typically considered objective are significantly impacted by arbitrary technical decisions made by those creating them.

Related Posts

By MrHonner, 8 years ago

Geometry Testing

Regents Recap — June 2016: Are These Figures Congruent?

Given the congruent triangles below, is the statement “Triangle ABC can be proved congruent to triangle ZYX” true, or false?

I imagine most will say that the statement is false, and argue that the correspondence of the triangles is incorrect. That is, segment AB is not congruent to segment ZY, and so on. I think this is a reasonable response.

However, a substantial part of me believes the statement is true. “Triangle ABC” references an object, as does “triangle ZYX“. These two objects are indeed congruent. Thus, how can it be said they can’t be proved congruent?

In other words, I don’t believe the statement “Triangle ABC can be proved congruent to triangle ZYX” entails a binding correspondence in the way that the statement

$\Delta ABC \cong \Delta XYZ$

does.

I was thinking about this because of this question from the June 2016 Common Core Geometry Regents exam.

According to the rubric, the correct answer is (3) reflection over the x-axis. The most common incorrect response, of course, was (1) rotation. But I’m not certain it’s really incorrect. I don’t think anyone would get this question wrong based on my objection, but since the question is designed to entice students to say rotation, I think it deserves some scrutiny.

Related Posts

By MrHonner, 8 years8 years ago

Testing

Follow Mr Honner