Regents Recap — June, 2017: Trouble with Dilations (and Logic)
The emphasis on transformations in Common Core Geometry has proven to be a challenge for the creators of the New York State Regents. Here’s the latest example.
This is a tricky question. So tricky, in fact, that it tripped up those responsible for creating this exam.
Dilation is a similarity mapping (assuming, as we do, that the scale factor is non-zero), and translation is a congruence mapping. Thus, any composition of the two will be a similarity mapping, but not necessarily a congruence mapping. So in the above question, statement II will always be true, and statements I and IV are not always true.
Statement III requires closer attention. Under most circumstances, translations and dilations map lines to parallel lines, and so the same would be true of their compositions. However, if the center of dilation lies on a given line, or the translation is parallel to the given line, then that line will be mapped onto itself under the transformation.
This means that the answer to this test question hinges on the question, “Is a line parallel to itself?”
If the answer is yes, then statement III will always be true, and so (3) II and III will be the correct answer. If the answer is no, then statement III won’t always be true. and so (1) II only will be the correct answer.
So which is the correct answer? Well, that’s tricky, too. The answer key provided by New York state originally gave (3) as the correct answer. But several days later, the NYS Department of Education issued a memo instructing graders to accept both (1) and (3) as correct. Apparently, the state isn’t prepared to take a stance on this issue.
Their final decision is amusing, as these two answer choices are mutually exclusive: either statement III is always true or it isn’t always true. It can’t be both. Those responsible for this exam are trying to get away with quietly asserting that (P and not P) can be true!
Oddly enough, this wasn’t the only place on this very exam where this issue arose. Here’s question 6: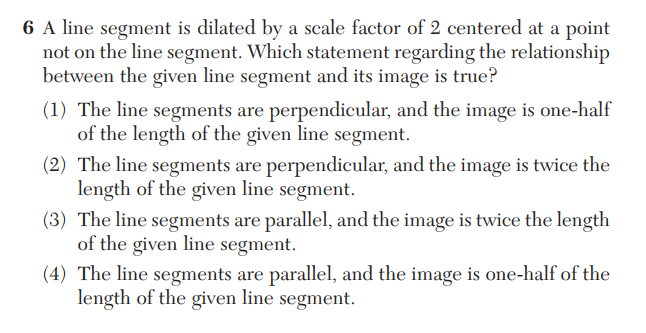
Notice that this question directly acknowledges that the location of the center of dilation impacts whether or not a line is mapped to a parallel line. It’s not entirely correct (a center’s location on the line, not the segment, is what matters) but it demonstrates some of the knowledge that was lacking in question 14. How, then, did the problem with question 14 slip through?
As is typical, the state provided a meaningless and generic explanation for the error: this problem was a result of discrepancies in wording. But there are no discrepancies in wording here. This is simply a careless error, one that should have been caught early in the test production process, and one that would have been caught if production of these exams were taken more seriously.
Related Posts
- Regents Recaps
- More Mathematical Misunderstanding
- The Worst Regents Question of All Time
- Another Embarrassingly Bad Math Exam Question
- Algebra is Hard
11 Comments
Amy Hogan · July 20, 2017 at 12:03 pm
I like your explanation of the “discrepancies” here, Patrick.
In my mind, this comes down to an issue of incomplete information regarding the transformation. To me, clear communication of a dilation includes a scale factor AND a center. Blanket statements about transformations like this make it so that the test-taker is forced to imagine what those things _might_ be.
As we know, dilations don’t act in a consistent manner in the plane. Negative scale factors, a scale of 1 (perhaps trivial, but still a possibility under this generic language), something greater than zero but less than one, etc. will produce different results. Likewise, varying centers of dilation (on vertex, on line segment, inside triangle, outside triangle,…) will give some interesting and very different results. And, most definitely, as you mentioned, where that center of dilation is will influence whether or not the image’s segments are parallel to its pre-image.
The lack of this information in this problem is equivalent to hand-waving on the part of the test committee. “Well, you know, a dilation.” The geometry student is going to have to fill in the blanks. A creative geometry student is going to fill in those blanks creatively. And in a timed test, why is this necessary? Adding more specific information about a scale and center wouldn’t inhibit the assessment of the question’s intent, as far as I can tell.
It shouldn’t be the test-takers job to both write the question and answer it. Perhaps in the future, students will have Mad Lib Regents questions: [Geometric Figure] is the image of [Geometric Figure] after a [Transformation] followed by a [Transformation]. Which statement(s) will [Always, Sometimes, Never] be true? 😛
MrHonner · July 20, 2017 at 5:17 pm
I think it’s appropriate, and important, to push students to think about abstract mathematical concepts (an arbitrary dilation, for example). When specified properly, these various transformations do have general properties that can be reasoned about. To me, the only problem with “incomplete information” is that of incomplete understanding in the minds of those who oversee this exam.
Jerome Dancis · July 20, 2017 at 2:03 pm
The real question is: “Is a line parallel to itself?”. As noted, this depends on the definition. Hopefully, there is a glossary or list of definitions on the state website.
If the definition excludes a line being parallel to itself, then being parallel would not be a transitive property. Boo. This would then require the awkward statement:
Theorem. If Line A is parallel is to Line B, which in turn is parallel to Line C, then Line A is parallel to Line C (except when Lines A and C are the same).
MrHonner · July 20, 2017 at 5:10 pm
Jerome-
There is no official list of definitions provided by the state. The closest approximation to that are the curricular materials provided by EngageNY (NY’s official unofficial curriculum), and those materials support the claim that a line is not parallel to itself.
Jerome Dancis · July 21, 2017 at 10:37 am
Mathematics is written literally and explicitly. Merely, supporting a claim is not Mathematics.
Emily DeSantis, a spokeswoman for the NYSED, mentioned that the Geometry Regents Exam is written and graded according to the concepts included in geometry, specifically cluster G.SRT.B, ( in her recent email to ABC News). Yes, the Geometry Regents Exam made national news; albeit just the grading of one problem.
Does this cluster G.SRT.B not provide definitions?
Does this cluster G.SRT.B or EngageNY include:
Theorem. If Line A is parallel is to Line B, which in turn is parallel to Line C, then Line A is parallel to Line C (except when Lines A and C are the same).
or
Theorem. If Line A is parallel is to Line B, which in turn is parallel to Line C, then Line A is parallel to Line C.
MrHonner · July 21, 2017 at 11:55 am
Jerome-
You are welcome to inspect for yourself.
The G.SRT standards are here, and I’ve create a Google search of Engage NY materials for “parallel line theorem” here.
Max McMahon · July 23, 2017 at 8:38 pm
Though it’s not part of the geometry standards, they do touch on the idea that parallel lines and the same line are distinct entities through standard form systems of linear equations (ax + by = c and a’x + b’y = c’). They define a system of linear equations that has no solution as parallel lines (a/a’ = b/b’ only) and a system of linear equations that has infinite solutions as the same line (a/a’ = b/b’ = c/c’).
MrHonner · July 24, 2017 at 8:48 am
Yes, good point. This is another situation where consequences of the definition of parallel pop up: are the graphs of two linear equations with no common solution “parallel”, or “parallel and distinct”?
Max McMahon · July 23, 2017 at 8:18 pm
At east it isn’t the 2008 8th grade exam where they gave points for doing a question wrong and not for doing it right…
MrHonner · July 24, 2017 at 8:34 am
Max-
I’m not familiar with that problem. Do you have a link?
But it reminds me of this Regents question from 2011, where the student’s score decreased for being less incorrect.
Max McMahon · July 24, 2017 at 4:39 pm
I can’t find the link (though I kept the scoring guide, it’s in storage somewhere) but the problem was “solve and check: 3(p + 6) = 5p + 4” with separate scorings for the ‘solve’ and the ‘check.’ The ‘solve’ part was fine, but the check was horrific (the most horrific part being how difficult it was to find someone at the site or headquarters who even understood why it was a problem).
On the rubric, they completely ignored the substitution of 3(7 + 6) = 5(7) + 4.
The first line was 21 + 18 = 35 + 4. The graders at my site were almost universally not giving any points for putting 3(13) = 35 + 4. It would have been one thing (still frustrating but logistically understandable) if they gave credit for doing it both ways, but, for over two days, literally nobody i spoke to understood that using the distributive property (rather than the order of operations) to check this problem was flat out wrong. Finally I ended up FAXING an explanation as to why it was wrong, demonstrating that if you mess up the distribution by only multiplying the variable, you’ll get that the answer is 1 and not 7, and a subsequent check (making the same mistake) would confirm that one was the correct answer. In the afternoon of the 3rd (and final) day of grading, somebody at headquarters finally conceded that it was flat out wrong and that they were giving points to kids who got it wrong but not those who got it right, but told me that it was too late to do anything about it. I was a first year teacher so didn’t want to piss anybody off any more than I had already, but my one of my biggest regrets from now 10 years of teaching is that I didn’t fight even harder (or just gone to the News or Post). But the worst part wasn’t that the rubric was wrong to begin with, it was how few people, including fellow teachers, who even partially understood that there was even a problem in the first place…