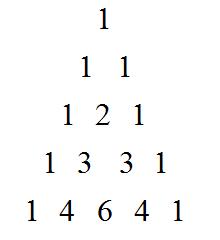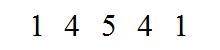Inspired by the recent increases in fares for public transit in NYC, I used Geogebra to put together a little graph to compare the various consumer options.
The solid red line represents the unlimited monthly card, and this costs $104 regardless of how many times it is used. The solid blue line represents a pay-per-ride strategy, plotting the total cost against the number of rides purchased.
These lines intersect at the point (49.46 , 104), meaning that the two plans are equivalent if one rides 49.46 times per month. Graphically, you can see that pay-per-ride is a better value for less than 49.46 rides (it’s lower than the red line), and is a worse value above that number (higher than the red line).
The dotted lines factor in the discount many New Yorkers enjoy by using pre-tax dollars to purchase transit cards. The use of pre-tax dollars saves you whatever you would have paid in income taxes on that amount: for New York City residents, the combination of federal, state, and city taxes is around 35% for typical earners. The discount affects both plans equally, so the point of intersection of the two dashed lines occurs at the same number of rides as the POI of the solid lines.
An astute observer might wonder why the equation of the solid blue line is not y = 2.25 x. While the fare is indeed $2.25 per ride, by pre-purchasing rides in bulk you receive a 7% discount. This changes the effective fare per ride, which is taken into consideration in the above graph. A trip over to the metrocard bonus calculator might shed some light on the subject.