There must be a good geometry problem in here somewhere.
Appreciation Resources
Gumdrop Solids
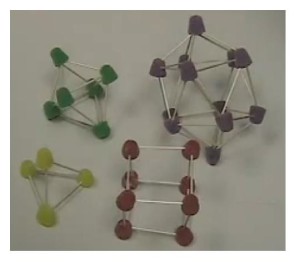This is a nice little video demonstrating how to use gumdrops and toothpicks to create Platonic Solids.
http://www.youtube.com/v/5QgIJOy7T7Y
A Platonic Solid is basically the 3-dimensional version of a regular polygon. A regular polygon is a 2-dimensional figure whose sides and angles are all congruent. A Platonic Solid is a 3-dimensional figure whose faces are all congruent, and the faces are put together at every vertex in the same way.
The most common example is the cube: it has six identical faces (squares), and each vertex is formed by putting three squares together at right angles to each other.
Quite remarkably, there are only five Platonic Solids: the tetrahedron, the cube, the octahedron, the icosahedron, and the dodecahedron. There are many other solids with interesting properties, but only five that satisfy the above conditions.
Our video-maker wasn’t ambitious enough to construct a dodecahedron. Or maybe she just didn’t have enough gumdrops.
Appreciation Challenge
Coffee and Cream – An Elegant Solution
My colleague, Scott Matthews, offered a very elegant solution to the Coffee and Cream mixture problem in response to the straight-forward solution I put forward. As he commented:
In the end, both cups have the same amount of liquid. Therefore, by the simple law of displacement, the amount of cream in the coffee is the the same as the amount of coffee in the cream.
This is a really nice way to think about the problem. In the end, the “coffee” cup contains the same amount of liquid as it started with. Therefore, any cream that ends up in the “coffee” cup had to displace some coffee.
Where did that coffee go? It ended up in the “cream” cup! Here’s a visual interpretation.
Thanks, Scott, for the elegant solution!
Related Posts
Application Appreciation
Daylight Saving Time is Mathematically Illogical
I’ve always found daylight saving time confusing, and now I know why: mathematically, it doesn’t make sense.
As summer turns to winter and our part of the Earth spends less time in the sun, the length of the day contracts. This kind of transformation is known as a dilation–a shrinking or stretching of something.
The yellow part of the graph represents the amount of sunlight per day. This part dilates, while the times-of-day on the clock stay fixed.
In order to increase the amount of productive daylight, we translate the times-of-day. Naturally, this doesn’t change the amount of available sunlight; it simply shifts the clock-times so that more of that sunlight occurs during preferred times-of-day .
Thus, the new day looks like this.
Essentially, daylight saving time tries to counteract a dilation with a translation. But mathematically, the way to truly counteract a dilation is with another dilation! Thus, the mathematical logic of daylight saving time is faulty.
Now that I fully understand the source of my confusion, I can rest easier. And for an extra hour!
Appreciation Challenge Geometry
Geometry Photography Competition
The New Scientist magazine has a monthly photo contest, and the theme this month is Geometry!
I’m thinking of submitting my picture of Parabolas in Nature, but probably not my picture of my imperfect division of a squash.
The contest ends November 30th, at midnight. The winner and select runners-up will be posted on the New Scientist website. All the details can be found here:
http://www.newscientist.com/article/dn19684-photo-competition-geometry.html
So keep your eyes open and your camera at hand–there’s a lot of Geometry out there!