Gumdrop Solids

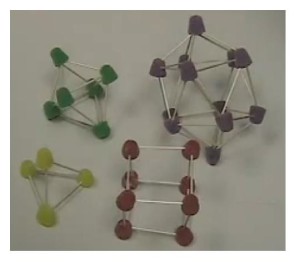This is a nice little video demonstrating how to use gumdrops and toothpicks to create Platonic Solids.

http://www.youtube.com/v/5QgIJOy7T7Y

A Platonic Solid is basically the 3-dimensional version of a regular polygon.  A regular polygon is a 2-dimensional figure whose sides and angles are all congruent.  A Platonic Solid is a 3-dimensional figure whose faces are all congruent, and the faces are put together at every vertex in the same way.

The most common example is the cube:  it has six identical faces (squares), and each vertex is formed by putting three squares together at right angles to each other.

Quite remarkably, there are only five Platonic Solids:  the tetrahedron, the cube, the octahedron, the icosahedron, and the dodecahedron.  There are many other solids with interesting properties, but only five that satisfy the above conditions.

Our video-maker wasn’t ambitious enough to construct a dodecahedron.  Or maybe she just didn’t have enough gumdrops.