Through Math for America, I am part of an on-going collaboration with the New York Times Learning Network. My latest contribution, a Test Yourself quiz question, can be found here:
http://learning.blogs.nytimes.com/2011/05/11/test-yourself-math-may-11-2011/
This question is based on the recent takeover of the LA Dodgers by Major League baseball. Just how bad is the situation for Los Angeles!
Here’s another introduction to the one-cut challenge, this time with quadrilaterals. This is another activity for students of all ages from my Fun with Folding series, and you can try this out even if you haven’t fully explored the one-cut challenge with triangles.
Recall that in the one-cut challenge, your task is to produce the given shape as a cut-out using only straight folds and asingle straight cut.
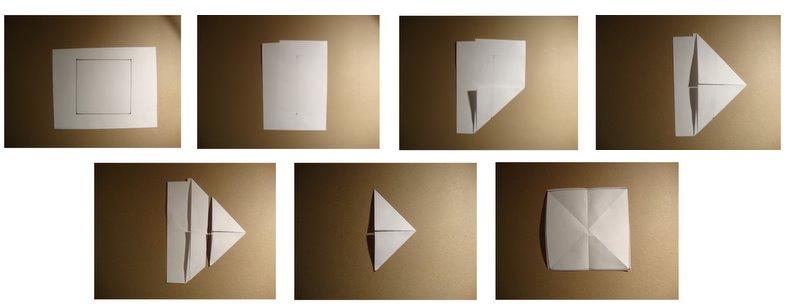
Let’s start with a square. One simple approach is to fold the square in half, then fold the top and bottom halves up along the vertical side.
This same approach also works with rectangles.
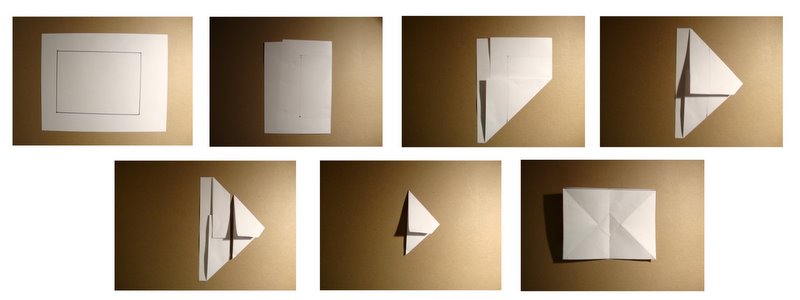
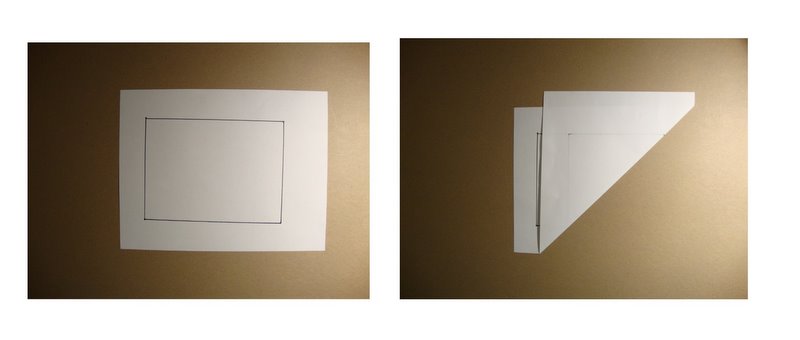
A second approach with the square involves fewer folds. First, fold along the main diagonal, then go from there.
In addition to being a more efficient solution, and raising the general question “What is the minimum number of folds needed to produce the shape?”, this approach produces some confusing results when applied to a rectangle!
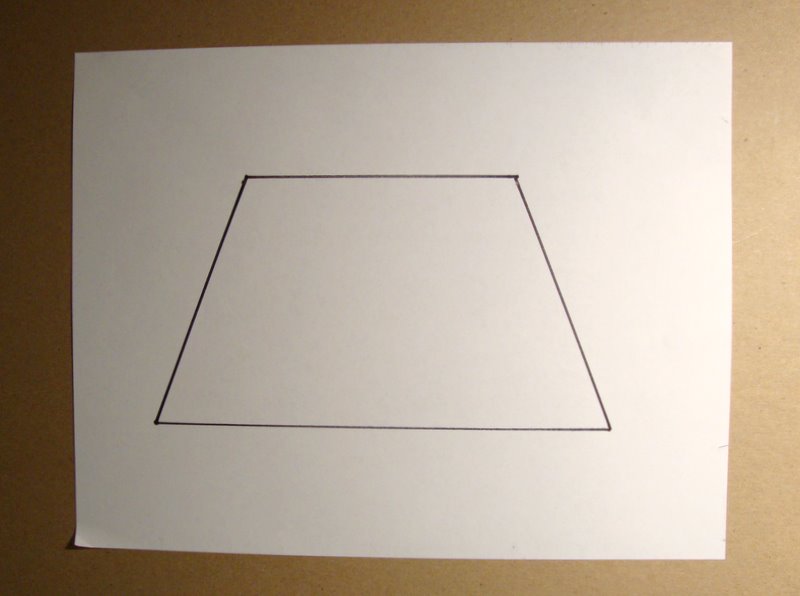
Another easy quadrilateral to work with is the isosceles trapezoid.
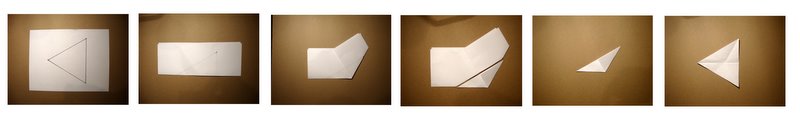
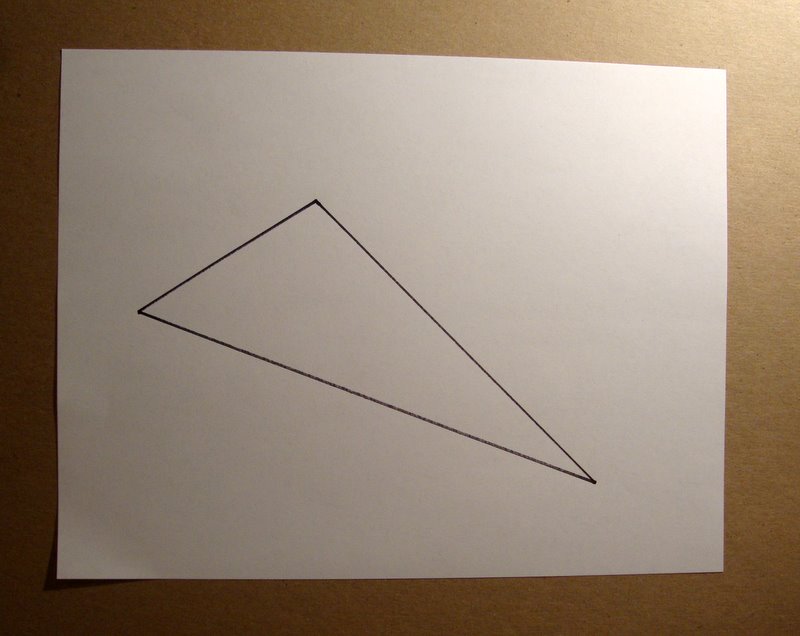
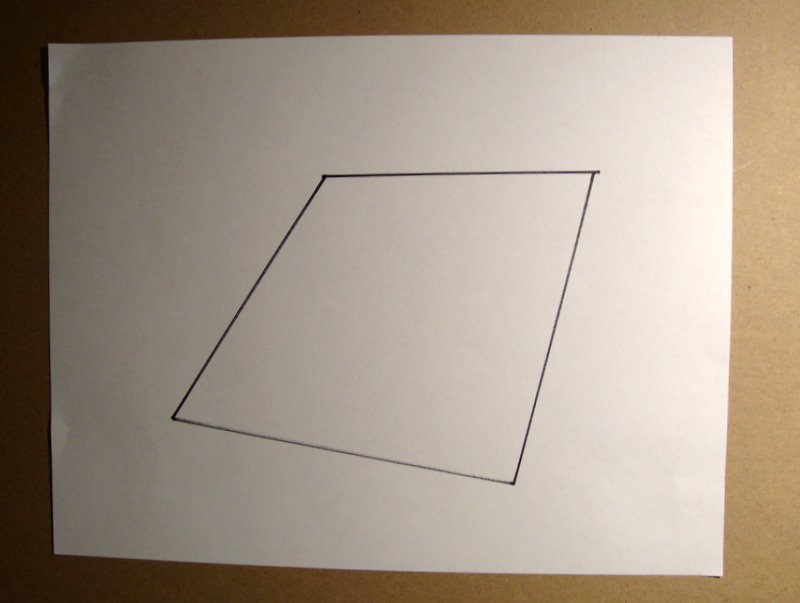
But watch out: an arbitrary quadrilateral poses quite a challenge!
Happy folding!
Have more Fun With Folding!
Here’s an introduction to the one-cut challenge using triangles, from my Fun with Folding series, suitable for students of all ages (including teachers!). This is a rich, compelling problem that touches on a lot of sophisticated ideas in geometry, but is simple enough to start playing around with right away.
The one-cut challenge is as follows: given a shape made up of connected straight line segments (i.e. a polygonal chain), can you produce the shape as a cut-out using only straight folds and a single straight cut?
A good place to start is with an equilateral triangle. This is a fairly easy problem to solve, given the inherent symmetry in the figure. Fold across any line of symmetry to produce a new figure that looks like two line segments meeting at an angle. Fold those together along their vertex, and cut!
The next step is trying this with an isosceles triangle, whose single line of symmetry still allows this approach to work.
Now the kicker: try it out on a scalene triangle! No more lines of symmetry, and all of the sudden this is a pretty challenging problem!
Happy folding!
Have more Fun With Folding!
While on the beach one day, I found a triangular rock. So I started on some beach relief.
Then I iterated.
And I iterated again.
And I continued to iterate, until the whole beach was one big Sierpinski Triangle!
This website provides running tallies on several world-wide statistics:
Data on Population, Energy, Economics, and Health are all constantly “updating”, brought you you by the Real Time Statistics Project.
In addition to the obvious questions one could ask, like “At what point will the world’s population grow to over 10 billion?” or “When will the earth run out of oil?”, there are interesting meta-questions like “Where do these models come from?” and “What assumptions are being made to calculate the amount of money spent on weight-loss programs?”.
Another nice resource to play around with!