I offered a classic mixture problem–Coffee and Cream–and I suppose it is appropriate that the poll results show a mixture of opinions. Although one answer clearly seems to be the favorite, there is still strong support for the other two options. I offer here one approach to solving this classic conundrum.
We begin by adopting a powerful problem solving strategy, one that when used incorrectly can lead to disaster, but when used properly, can turn hard problems into simple ones: we make a wish.
In the statement of the Coffee and Cream problem, no real mention of measurements were made, and, since all the results are ratios anyway, we can wish for whatever starting amount we want. So, let us wish that each cup contains 10 spoonfuls, and proceed from there.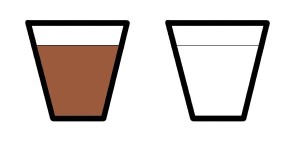
So there are 10 spoonfuls of coffee on the left, and 10 spoonfuls of cream on the right. We take one spoonful of cream, and add it to the coffee.
There are now 11 spoonfuls of liquid in the cup on the left: 10 spoonfuls of coffee and 1 spoonful of cream. Thus, the cup on the left is 1/11 cream and 10/11 coffee. Now we mix.
Mixing doesn’t change the amounts of coffee and cream in the cup: the cup on the left is still 1/11 cream and 10/11 coffee. So we take one spoonful of that, and add it to the cream.
The cup on the left once again has 10 spoonfuls of liquid, and that liquid is 1/11 cream and 10/11 coffee. So of the liquid on the left, 1/11 of 10 spoonfuls, or 10/11 of a spoonful, is cream. In other words, there is 10/11 of a spoonful of cream in the coffee.
Now the cup on the right also again has 10 spoonfuls of liquid in it. How much of that is coffee? Well, the only coffee in the cup on the right came from the one spoonful of mixture we just added. And that mixture is 10/11 coffee! 
Whether we mix it up or not, there is now 10/11 of a spoonful of coffee in the cream.
Related Posts
The New Scientist magazine has a monthly photo contest, and the theme this month is Geometry!
