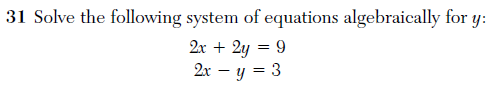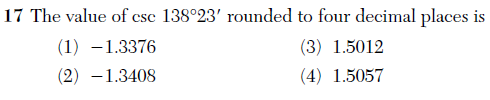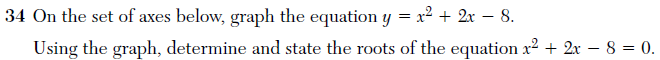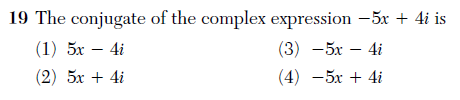August 2012

Here is another installment from my review of the June 2012 New York State Math Regents exams.

Below are a few examples of what I consider “bad” questions.  “Bad” here might mean poorly worded, poorly conceived, or irrelevant.  In addition, there is an example of a question with a problematic rubric.

First, a type of problem that occurs regularly, one that is a pet peeve of mine.  From the Algebra 2 / Trig exam:

The concept of “middle term” is artificial and depends entirely on how one chooses to evaluate the given expression.  This question does not test an authentic mathematical skill; it tests how well a student executes one particular method of evaluating this particular expression.

Next, an example of a poorly-phrased question, one that confuses mathematical terminology.  From the Integrated Algebra exam:

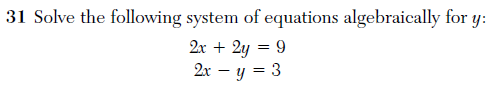
To “solve” a system of equations, one must find the ordered pairs that satisfy the given equations.  Apparently this question wants only the y-values of those solutions, but the phrasing confuses what it means to “solve a system” and to “solve an equation”.

Students can probably figure out what the question-writer wants to hear in this case, but the lack of precision will only exacerbate confusion about the word “solve”.

Here’s a problem on the Algebra 2 / Trig exam that is simply irrelevant.

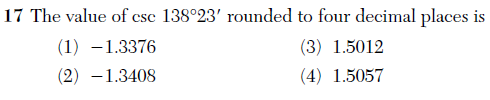
This question tests one thing, and one thing only:  knowledge of an arcane and largely irrelevant notation, namely, degree-minute-second representation of angles.  Would anyone outside the nautical or astronomical worlds consider this even remotely valuable?

Lastly, this question from the Integrated Algebra exam is formulated in a reasonable way, but the official scoring guide poses some unnecessary problems.

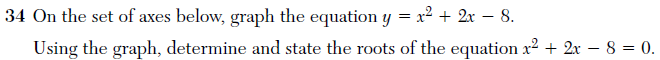
This question asks the student to graph an equation and then, using the graph, determine and state the roots of the equation.  The correct answer is “2 and -4”, and with appropriate work, is worth three points.

However, if the student gives the answer “(2,0) and (-4,0)”, the student can only earn two out of the three points.  So if the student gives the coordinates of the points where the graph crosses the x-axis, rather than names the “roots” of the equation, there is a one-third deduction.

While I believe that the distinction between roots and points is important, losing one-third credit seems seems unnecessarily punitive here.  If we want to test student’s knowledge of vocabulary, there are better ways to do it than by sneaking it in at the end of an involved algebra problem.

Moreover, since the question requires that the student use the graph, the student is already being forced to interpret the problem in a geometric context.  Penalizing them for thinking of the roots geometrically, then, doesn’t quite seem fair.

Here is another installment from my review of the June 2012 New York State Math Regents exams.

Mathematically erroneous questions consistently appear on these exams.  Here are two recent examples, both from the Algebra 2 / Trigonometry exam.

According to the scoring key, the correct answer is (4).  This would be the correct answer if the angle were given as -50 degrees.  Notice, however, that no degree symbol is present.  This means the angle is actually -50 radians.  In degrees, -50 radians is equivalent to roughly -2864.8 degrees, which itself is equivalent to roughly 15 degrees.  Thus, the actual correct answer is (3).

The above problem could be considered a typo (although no correction was ever issued), but the most erroneous Regents questions demonstrate a real lack of mathematical understanding on the part of the exam creators.   Consider the following question on complex numbers.

None of these answers are correct.

The exam writers believe that (3) is the correct answer.  Given a complex number a + bi,  the conjugate is indeed a – bi, provided that a and b are real numbers.  But x is a variable, and there is no reason to assume that x has to be a real number.  If x = i, for example, (3) is not the complex conjugate of  -5x + 4i.  In this case, the conjugate of the original expression is i, while (3) evaluates to – 9i.

As emphasis on standardized exam performance continues to grow, a few points here or there can make a big difference in the lives of students, teachers, and schools.  The consistent appearance of erroneous mathematics on these exams calls into question their validity as a measurement of “student achievement”.

Below is a collection of posts analyzing the June 2012 New York State Math Regents exams.

The purpose of these analyses is to bring critical attention to the quality and utility of these standardized exams, which play an increasingly substantial role in today’s educational environment.

But, as the role of standardized tests continues to grow in the evaluation of students, teachers, schools, and districts, one question rarely gets asked:  “Are these tests any good?”.

Analyses of June 2012 New York State Math Regents Exams

June 2012 Math Regents Exams — Mathematically Erroneous Questions

June 2012 Math Regents Exams — Poorly Constructed Questions

June 2012 Math Regents Exams — Unscaled Graphs

June 2012 Math Regents Exams — Throwing Darts

June 2012 Math Regents Exams — Spot the Function

June 2012 Math Regents Exams — Some Improvement

Click here to see recaps of other New York State Math Regents exams.