My students and I experimented a bit with 3D printing at the end of the year (as part of my always-do-something-new strategy). This (5,3) Torus knot was one of our successes.
I see a lot of possibility for 3D printing in my classrooms next year, and I’m looking forward to thinking and learning more about it.
I am huge fan of Desmos, the free online graphing calculator. I use it almost every day in my classroom: to sketch simple graphs, demonstrate mathematical relationships, and dynamically explore mathematical situations. And like most worthy instructional technologies, it’s really a learning technology: it’s easily accessible to students as well as teachers..
As far as technology goes, Desmos works very well. But some of my favorite mathematical questions arise when technology does something we don’t expect.
For example, here’s the graph of 
But look what happens when you zoom in around the hole:
At a very small scale, some very curious behavior emerges!
Now, it’s not the function here that’s behaving strangely: its behavior is well-understood. It’s the mathematical technology that is behaving strangely, as it tries to represent the function.
Lots of interesting questions emerge from such anomalies, and these are great questions for students to explore. In doing so, they’ll not only learn some mathematics and some computer science, but they’ll also develop a healthier relationship with technology, by learning to understand how it does what it does, and perhaps more importantly, what it doesn’t do. I explore this theme in greater depth in my talk “When Technology Fails“.
You can find more of my work with Desmos here.
Related Posts
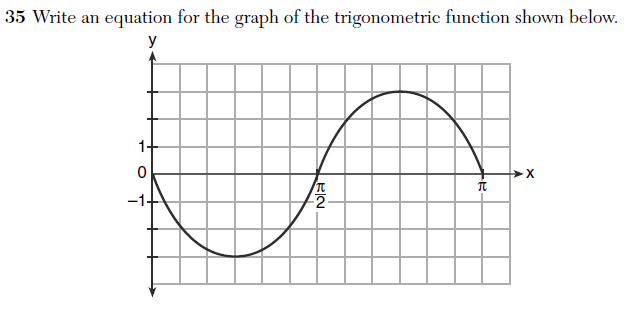
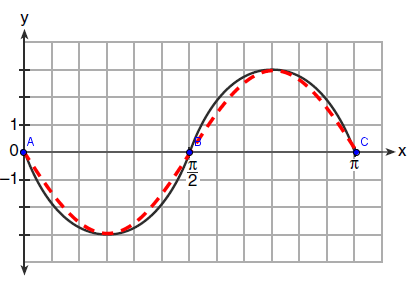
I have taught in a variety of physical settings, from old-school classrooms with desks bolted to the floor to modern environments that synthesize collaborative space with individual work stations. In these various spaces I’ve successfully integrated all kinds of technology into teaching and learning: smartboards, projectors, clickers, laptops, sensors, calculators, and the like.
As far as I’m concerned, sometimes all a class needs is one good problem written on the board, but overall I consider myself to be a technology-positive teacher. I like to try to new things and I do my best to use what’s available.
But without question, this is the single most valuable piece of instructional technology I’ve encountered.
I started teaching in a classroom full of these desks several years ago, and I’d hate to have to teach without them. They are light, easy to move around, and extremely flexible when it comes to grouping.
It’s so easy to transition from pairs to fours
that students do it without prompting. When the situation calls for more collaboration, they simply rearrange themselves. If they want to merge into another group, they do it.
I love the wealth of tools that are available to math teachers now–Geogebra, Desmos, Wolfram Alpha, Sage, and others–but if I had to choose just one thing to have in my classroom, these desks would be it.
Here is a fully formed Real Analysis course freely offered online:
http://www.webskate101.com/webnotes/home.htmld/home.html
The course includes a sequence of 60 classes with notes, a fully hyper-linked Analysis textbook, and a set of homework assignments.
This resource comes from John Lindsay Orr, a mathematician at the University of Nebraska.
It seems as though this WebNotes platform might be available for other teachers to create their own online courses.
This is a great resource: thousands of free video lectures on a variety of topics from CosmoLearning.
There are over a thousand videos on mathematics, including full courses in Single-Variable Calculus, Vector Calculus, Differential Equations, Trigonometry, Statistics, and the History of Mathematics.
There are also standalone videos on a wide variety of topics like Topology, Probability, and Algebra.
In addition to Mathematics, there are thousands of other videos ranging from Anthropology to Veterinary Medicine!