Teaching

3D Printing Geometry Teaching

Cavalieri’s Principle and 3D Printing

These 3D printed objects served as an excellent starting point for a classroom conversation on Cavalieri’s Principle.

Each shape above is an extrusion of the same square. In the middle, the square moves straight up; at left, the square travels in a complete circle from bottom to top; and at right, the square moves along a line segment and back.

The objects all have the same height. Since at every level they have the same cross-sectional area, by Cavalieri’s Principle they all have the same volume!

Cavalieri’s Principle is a simple but powerful idea, and one that can be easily demonstrated around the house. Here are some other examples using CDs and CD cases.

By MrHonner, 10 years10 years ago

Challenge Resources Teaching

Rosenthal Prize Application Workshop

I recently participated in a workshop hosted by the Museum of Mathematics about the Rosenthal Prize for Innovation in Math Teaching. The Rosenthal Prize invites classroom teachers to submit outstanding, fun, creative, and engaging math lessons: the author of the best lesson receives $25,000, and other noteworthy submissions are honored as well.

The purpose of the workshop was to help prospective applicants understand the submission, revision, and judging process for the prize. The workshop panel included the directors of the museum, past judges, and three former winners of the Rosenthal Prize (including myself).

The video is embedded below, or you can watch on YouTube here.

Please spread the word about the Rosenthal Prize: it’s rare to have such incentive to build and share creative, engaging mathematics lessons!

By MrHonner, 10 years10 years ago

Geometry Teaching

Sum of Angles in Star Polygons

Futility Closet recently posted a nice puzzle about the sum of the angles in the “points” of a star polygon.

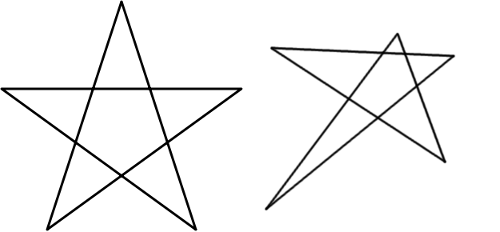
It’s easy to show that the five acute angles in the points of a regular star, like the one at left, total 180°.

Can you show that the sum of these angles in an irregular star, like the one at right, is also 180°? (link)

A clever proof is shown, but what I would consider the standard proof is clever, simple, and beautifully generalizable.

Consider the star pentagon below.

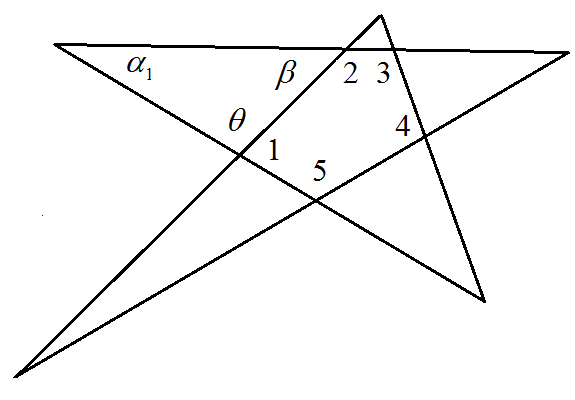By the exterior angle theorem, we have

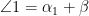
$\angle 1 = \alpha_1 + \beta$

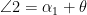
$\angle 2 = \alpha_1 + \theta$

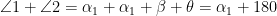
$\angle 1 + \angle 2 = \alpha_1 + \alpha_1 + \beta + \theta = \alpha_1 + 180 \textdegree$

where the last equality follows from the triangle angle sum formula.

Do this for each of the five “points” and sum the equations

$2( \angle 1 + \angle 2 + \angle 3 + \angle 4 + \angle 5) = \sum \alpha_i + 5 \times 180 \textdegree$

Now, the sum of the interior angles of any pentagon, regular or not, is 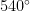$540 \textdegree$ , so this becomes

$2 \times 540 \textdegree = \sum \alpha_i + 5 \times 180 \textdegree$

And so, a little arithmetic gives us

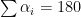
$\sum \alpha_i = 180 \textdegree$

For stars of this type, where the points are formed by intersecting two sides of an n-gon that are separated by exactly one side, this method generalizes beautifully. The above equation becomes

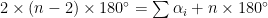
$2 \times (n-2) \times 180 \textdegree = \sum \alpha_i + n \times 180 \textdegree$

which simplifies to

$\sum \alpha_i = (n-4) \times 180 \textdegree$

In particular, we see that when n = 5, we have that 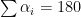$\sum \alpha_i = 180 \textdegree$ . And one of the best things about having a formula like this is asking questions like “What happens when n = 4?” and “What happens when n = 3?”!

Now, there are other types of star polygons. For example, if you start with an octagon, extend the sides, and consider the intersections of two sides that themselves are separated by exactly two sides of the octagon, you get something that looks like this.

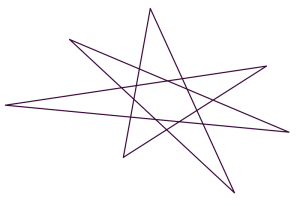
While the formula above doesn’t apply to this star, a similar technique does. The big difference is that, instead of the star’s points being attached an an n-gon (a pentagon, in the first example), this star’s points are attached to another star polygon! There are lots of fun directions to go with this exploration.

By MrHonner, 10 years9 years ago

Appreciation Teaching

2015 Rosenthal Prize

The Rosenthal Prize for Innovation in Math Teaching, presented by the Museum of Mathematics, aims to celebrate and promote the development of creative, engaging, and replicable math lessons. The winning teacher receives $25,000, and their lesson is shared freely by the Museum of Mathematics.

Applications for the 2015 Rosenthal Prize are now open. As a past awardee, I will be participating in an application workshop at the museum on Friday, May 1st. The purpose of the workshop is to help teachers with the application process by answering questions and providing feedback.

The workshop details, including registration information, are available here. For those unable to attend in person, the workshop will also be live-streamed.

I encourage interested teachers to consider applying for the Rosenthal Prize. It’s a unique and interesting challenge, and just participating in the process can be a growth experience.

By MrHonner, 10 years10 years ago

Statistics Teaching Testing

Regents Recap — January 2015: Questions with No Correct Answer

Here is another installment in my series reviewing the NY State Regents exams in mathematics.

This is question 14 from the Common Core Algebra exam.

Setting aside the excessive, and questionable, setup (do people really think about minimizing the interquartile range of daily temperatures when choosing vacation spots?), there is a serious issue with this question: it has no correct answer.

The student is asked to identify the data set that satisfies the following two conditions: median temperature over 80 and smallest interquartile range. No data set satisfies both these conditions. According to the diagram, the data associated with “Serene Shores” has the smallest interquartile range (represented by the width of the “box” in the box-and-whisker plot), but its median temperature (the vertical line segment in the box) is below 80.

The answer key says that (4) is the correct answer, but that data does not have the smallest interquartile range shown. Presumably, the intent was for students to evaluate a conditional statement, like, “Among those that satisfy condition A, which satisfies condition B?” But as written, the question asks, “which satisfies both condition A and condition B?” No set of data satisfies both.

Some may consider this nitpicking, but precision in language is an important part of doing mathematics. I focus on it in my classroom, and it is frustrating to see my work undermined by the very tests that are now being used to evaluate my job performance.

Moreover, this is by no means the only error present in these exams, nor is it the first example of errors in stating and evaluating compound sentences. If these exams don’t model exemplary mathematical practice, their credibility in evaluating the mathematical practice of students and teachers must be questioned.

By MrHonner, 10 years10 years ago

Follow Mr Honner