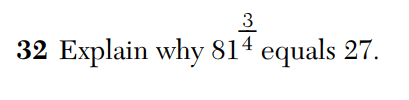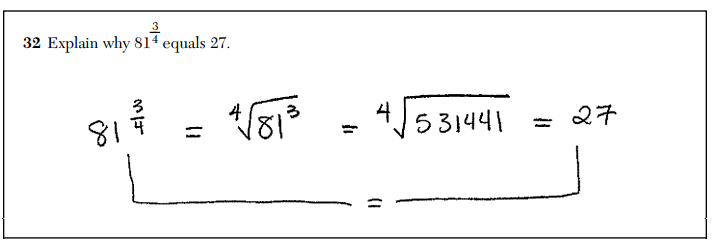Teaching

Regents Recap — January 2018: How Do You Explain That 2 + 3 = 5?

This has quickly become my new least-favorite kind of Regents exam question. (This is number 32 from the January, 2018 Algebra 2 Regents exam.)

What can you say here, really? They’re equal because they’re the same number. Here’s a solid mathematical explanation. Right?

Wrong.

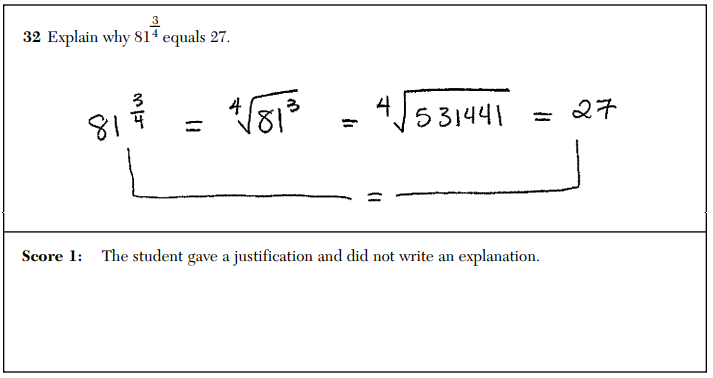
According to those who write the scoring guidelines for these exams, this is a justification, not an explanation. Because students were asked to explain, not justify, this earns only half credit.

This is absurd. First of all, this is a perfectly reasonable explanation of why these two numbers are equal. This logical string of equalities explains it all. This clear mathematical argument demonstrates what it means to raise something to the power 3/4.

Second, whatever it is that differentiates an “explanation” from a “justification” in the minds of Regents exams writers, it’s never been made clear to test-takers or the teachers who prepare them. A working theory among some teachers is that “explain” just means “use words”. Setting aside how ridiculous this is, if this is the standard to meet, students and teachers need to be aware of it. It needs to be clearly communicated in testing and curricular materials. It isn’t.

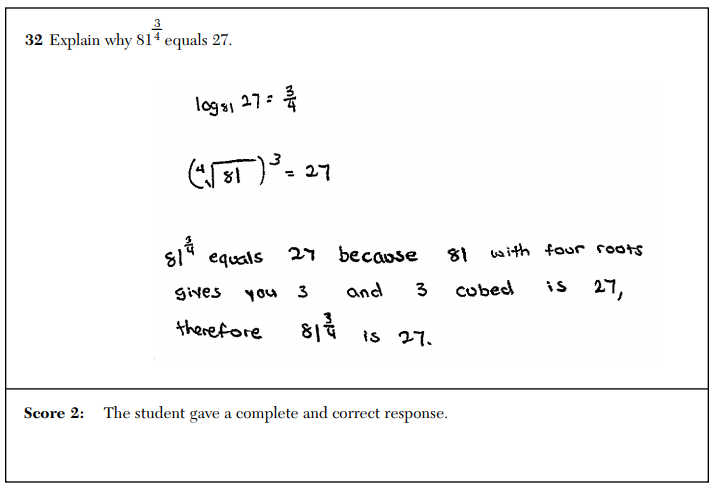
Third, take a look at what the the test-makers consider a “complete and correct response”.

In this full-credit response, the student demonstrates a shaky mathematical understanding of the situation (why are they using logarithms?) and writes a statement (“81 with four roots gives you 3”) that, while on the right track, is in need of substantial mathematical refinement. Declaring this to be a superior response to the valid mathematical argument above is an embarrassment.

These tests are at their worst when they encourage and propagate poor mathematical behavior. We deserve more from our high-stakes exams.

Related Posts

By MrHonner, ago

Regents Recap — January 2018: Is it Better to Justify or Explain?

On question 32 of the January, 2018 Common Core Algebra 1 Regents exam, students were asked to explain why a quadratic whose graph is given might have a particular set of factors. Here are two sample student responses from the state-produced Model Response Set.

On the left, the student says “Yes”, sets each factor to 0 and solves, and produces the roots x = -2 and x = 3. On the right, the student says “Yes, because the x-intercepts are (-2,0) and (3,0).”

One of these responses received full credit, the other half credit. I posted this to Twitter and invited people to guess.


According to the official scoring guide, the response on the right earned full credit: it is a “complete and correct response”. The response on the left earned half credit, because the student “gave a justification, not an explanation.”

It seemed as though the majority of respondents on Twitter favored the response on the left; a few even specifically said it offered a better “explanation” than the full-credit response. Many did choose the response on the right, especially those familiar with how New York’s Regents exams are scored.

To me, both answers are unsatisfying. The full-credit response offers an “explanation” but is devoid of justification: the student doesn’t make the connection between the x-intercepts and the roots. The half-credit response derives the roots algebraically, but fails to explicitly connect the roots to the intercepts. It’s hard for me to accept that one of these responses is substantially better than the other: both responses expect the reader to fill in an equally important gap.

It’s also hard for me to accept what counts as “explanation” here. Several teachers familiar with New York’s Regents exams commented that, in this context, “explain” simply means use words. And we’ve seen example after example of ridiculous “explanations” on these exams. It sends the wrong message to students and teachers about what constitutes mathematics, and since the message is transmitted via high-stakes exams, it can’t be ignored.

Related Posts

By MrHonner, ago

How Math (and Vaccines) Keep You Safe From the Flu — Quanta Magazine

My latest column for Quanta Magazine breaks down the mathematics of “herd immunity”. By vaccinating a critical percentage of a population against a disease, the potential spread of the disease through the population will proceed at a linear, not exponential, rate. This herd immunity can mean the difference between a handful of illnesses and a catastrophe.

We start by thinking about how rumors spread.

Let’s say you hear a juicy rumor that you just can’t keep to yourself. You hate rumormongers, so you compromise by telling only one person and then keeping your mouth shut. No big deal, right? After all, if the person you tell adopts the same policy and only tells one other person, the gossip won’t spread very far. If one new person hears the rumor each day, after 30 days it will have spread to only 31 people, including you.

So how bad could it be to tell two people? Shockingly bad, it turns out. If each day, each person who heard the rumor yesterday tells two new people, then after 30 days the rumor will have reached more than a quarter of the world’s population (2,147,483,647 people, or 231 − 1, to be exact). How can such a seemingly small change — telling two people instead of one — make such a big difference? The answer lies in rates of change.

A similar mathematical model can be used to understand the spread of disease. And by unpacking the mathematics behind the basic reproduction number of a disease, we can compute the critical cutoff for herd immunity.

Learn more by reading the full article, which comes with a classroom-ready worksheet and is freely available here.

By MrHonner, ago

Apple Pies are Delicious

“Cherry pie is delicious!” Nick said, with a big smile. “Apple pies are, too.” He was explaining his trick for remembering the formulas for the circumference and area of a circle. A bunch of his classmates nodded along, many who attended the same middle school as Nick. I didn’t quite get it.

Nick diagrammed it out for me.

“Cherry pie is delicious” –>  C \pi d  –>  C = \pi d

“Apples pies are, too” –>  A \pi r 2  –>  A = \pi r^2

Now, I don’t mind a good mnemonic now and then; I still sing the alphabet song, after all. But this struck me as extremely silly. These formulas get used all the time and they are deeply connected to many other important concepts. Relying on a memory trick creates a flimsy foundation for an important body of knowledge. I decided to show Nick just how flimsy.

The next day in class, I approached Nick. “You know, after thinking about it, I agree with you: apple pies are delicious.” He was pleased. But his smile quickly faded. He wrote something out in his notes. “Wait, that’s not right.”

“So apple pies are not delicious?” I asked.

“It’s ‘Cherry pie is delicious‘.” He showed me the formula.

“But apple pies are delicious, right?”

“Yeah, but that’s just not how it works.”

“This is kind of confusing”, I said. “Oh wait. Now I see. Apple pies are delicious too!” I wrote out A \pi r d 2, followed by A = \pi \frac{rd}{2}. “Perfect!”

“What?”

“See here,” I said. I wrote out  A = \pi \frac{rd}{2} = \pi \frac{r2r}{2} = \pi r^2. “You’re method works perfectly!”

Nick started scribbling more in his notebook. I quietly walked away.

Over the next few days I continued my demonstration. “Cherry pies are delicious, too!” I’d say. Or, “Apple pies are really, really delicious!” I might have even said something like “Some apple pies are to die for.”

My demonstration was successful. Maybe too successful. Nick got the area of circle wrong on the next test.

When I handed it back, he acknowledged my point with a combination of irritation and admiration. Nick never got the area of a circle wrong again. And we never had to talk about his Dear Aunt Sally again, either.

[No mathematical understanding was harmed in this story.]

By MrHonner, ago

The Problem with Pentagons — Scarsdale High School

I’m excited to be visiting Scarsdale High School, where I’ll be talking about the recent classification of pentagonal tilings of the plane. In my talk, The Problem with Pentagons, I’ll show how a math problem accessible to high school geometry students has a solution that ultimately spans centuries, cultures, and disciplines. The talk is based in part my recent article for Quanta Magazine.

I’m looking forward to meeting students and teachers, visiting some classes, learning more about Scarsdale HS, and talking about tilings!

Related Posts

 

By MrHonner, ago
Follow

Get every new post delivered to your Inbox

Join other followers: