While playing around with some light and shadows, I caught another quadrilaterals on my carpet:
I also blocked the light in such a way that it turned this trapezoid into a parallelogram.
Related Posts
Geometry Teaching
A New Solution to an Old Problem
This story makes me feel bad for every time I discouraged a student from a math research project because the topic was too well-known.
http://novinite.com/view_news.php?id=122377
A 19-year old Bulgarian student has solved the 2000-year old Problem of Appollonius in a new and unique way. It is the first new solution in 200 years, and only the fifth known solution overall.
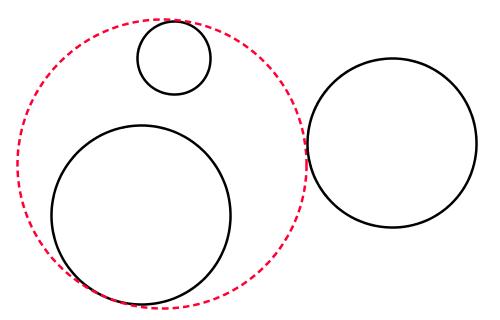
The Problem of Appolonius, essentially, is to construct (with straightedge and compass, only) a circle that is tangent to three given objects. Here is an example of an Appolonius Circle (in red) that has been constructed to be tangent to the three given circles (in black).
This story is nice reminder that sometimes the best thing to do as a teacher is get out of the student’s way!
Appreciation Geometry
CDs and Cavalieri’s Principle: Part 2
After demonstrating Cavalieri’s Principle with empty CD cases, I thought I’d do the same with the actual CDs.
Here we see a bunch of discs stacked up to make a right cylinder.
To compute the volume of this cylinder, it would be sufficient to know (a) the volume of one CD, and (b) the number of CDs in the stack. We would simply multiply the two together to get the volume.
The argument is less obvious, but essentially the same, regardless of how the CDs are stacked! So this “prism”
has the same volume as the original cylinder. Now, this object should also have the same volume
however some center-of-mass issues may foil our elegant mathematical demonstration.
Related Posts
Appreciation Geometry Photography
Math Photo: Solid Family
My niece and I were playing around with Magnaformers and we had just enough to create this family of geometric solids.
My niece argued that the figures should be ordered from smallest to biggest. Unimpressed by my argument that they were ordered smallest to biggest, if you considered the number of sides of each figure, she insisted on reordering them and we took another photo.
For someone who claims not to like math, my niece sure does enjoy playing around with mathematical ideas!
Related Posts
Appreciation Geometry
Foam Pyramids
More from my fun with folding series: after having fun making paper pyramids, I thought I’d try out foam board as a medium. As before, I began by connecting the midpoints of the sides of the triangle to form its medial triangle.
Then I scored the medial lines, bent, and taped!
A rotation gives a sense of how oblique this pyramid is.
My students and I enjoy exploring questions like “Will this procedure always produce a pyramid?” and “What other kinds of solids can be formed in this manner?”
Related Posts