Challenge

Challenge Teaching

Fun With Folding: Incenter

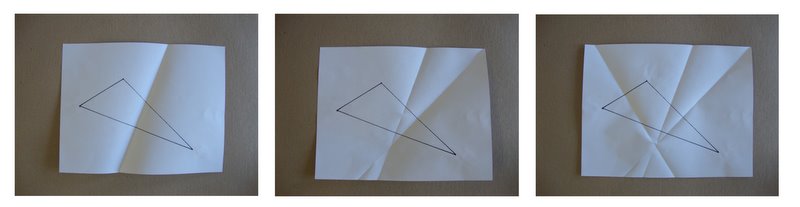
Here’s another entry from my Fun With Folding series: folding the incenter of a triangle! The incenter of a triangle has a lot of interesting properties, most of which are related to the fact that it is the center of the triangle’s unique inscribed circle.

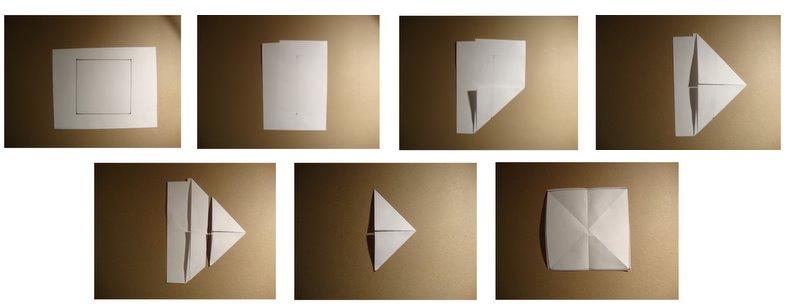
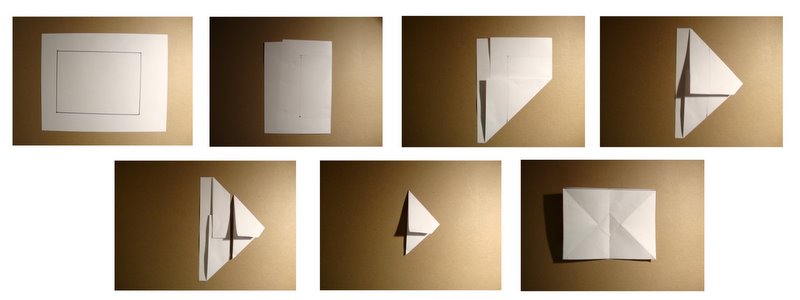
To fold your way to the incenter, start with an arbitrary triangle and use the angle bisector fold on each angle. (Click here to find instructions for the basic folds.)

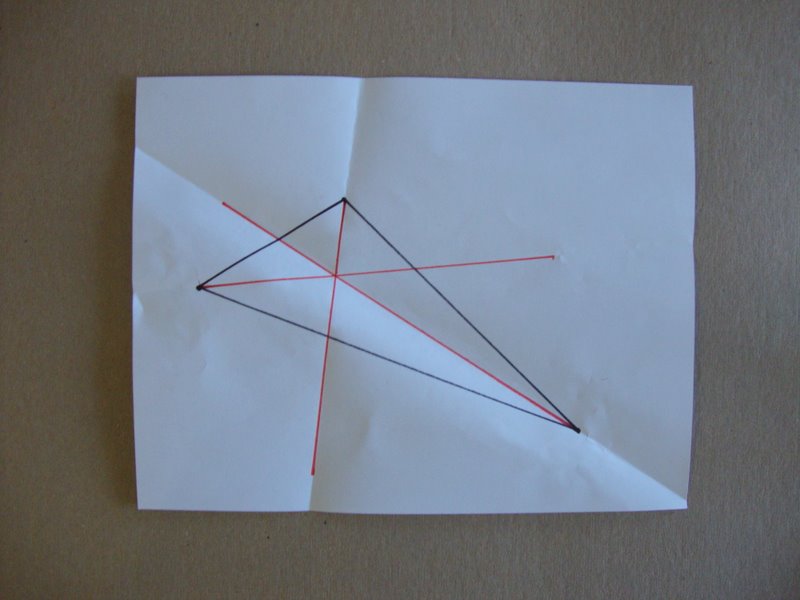
Like magic, all the angle bisectors intersect at the incenter!

Be sure to try some other fun mathematical activities with folding!

Have more Fun With Folding!

By patrick honner, 14 years6 years ago

Challenge Geometry

A Curious Geometric Limit

Inspired by Alexander Bogomolny (@CutTheKnotMath) and his curious geometric limit, I offer this trigonometric approach.

Consider the following diagram in which we have circle X of radius 3 centered at (3,0) and circle O of radius k centered at the origin. Call the intersection of the these circles in the first quadrant B. Let A be the intersection of circle O with the y-axis, and extend line AB until it intersects the x-axis at E. Our goal is to show that $\lim_{kto}OE=12$ .

Consider the diagram below.

Let $\angle{OXB} = \theta$ . Some simple angle chasing, using properties of isosceles triangles and right triangles, gives us that $\angle{OEA} = \frac{\theta}{4}$ .

Since AO = k by definition, we have $tan(\frac{\theta}{4}) = \frac{k}{OE}$ , and so OE = $\frac{k}{tan(\frac{\theta}{4}) }$ .

Since OB = k is the base of isosceles triangle OXB, we can drop an altitude from X to OB and find that $sin(\frac{\theta}{2}) = \frac{\frac{k}{2}}{BX} = \frac{\frac{k}{2}}{3}$ . This gives us $k = 6sin(\frac{\theta}{2})$ .

So OE = $\frac{k}{tan(\frac{\theta}{4}) }$ = $\frac{6sin(\frac{\theta}{2})}{tan(\frac{\theta}{4}) }$ . By the double angle formula for sine, we have

$sin(\frac{\theta}{2}) = 2sin(\frac{\theta}{4})cos(\frac{\theta}{4})$ ,

Making this substitution, and by writing tangent as the quotient of sine and cosine, we get

OE = $\frac{6sin(\frac{\theta}{2})}{tan(\frac{\theta}{4}) } = \frac{12sin(\frac{\theta}{4}) cos((\frac{\theta}{4}))}{\frac {sin(\frac{\theta}{4})}{cos(\frac{\theta}{4})} } = 12 {(cos(\frac{\theta}{4}))}^2$ .

Finally, since $\theta\to 0$ as $k \to 0$ , we have

$\lim_{k\to 0}OE = \lim_{\theta \to 0}12 {(cos(\frac{\theta}{4}))}^2 = 12\lim_{\theta \to 0} {(cos(\frac{\theta}{4}))}^2=12$ .

By patrick honner, 14 years8 years ago

Challenge Teaching

Fun With Folding: Basic Folds

Here’s a good place to start my Fun With Folding series. Below are the instructions for four basic folds: folding a linethrough two points; folding the midpoint of a line segment; folding the perpendicular bisector of a segment; and folding the angle bisector of an angle. Get these all down and you’ll be ready to have lots of fun with folding!

First, let’s fold a line between two points. The key here is to make sure that both points lie in the crease of the fold.

You can fold the midpoint of a line segment by folding in such a way that one endpoint of the line segment lies on top of the other. The two halves of the line segment should be right on top of each other, and just pinch the fold at the midpoint.

To fold the perpendicular bisector of a segment, perform the same steps as above for a midpoint, but just complete the crease!

The last basic fold is an angle bisector. Given an angle, make a fold that passes through the vertex of the angle so that the two sides of the angle lie on top of each other.

Once you’ve got these basic folds down, you’ve got everything you need to try some more advanced folding challenges!

Have more Fun With Folding!

By patrick honner, 14 years6 years ago

Challenge NYT Sports

Math Quiz: NYT Learning Network

Through Math for America, I am part of an on-going collaboration with the New York Times Learning Network. My latest contribution, a Test Yourself quiz question, can be found here:

http://learning.blogs.nytimes.com/2011/05/11/test-yourself-math-may-11-2011/

This question is based on the recent takeover of the LA Dodgers by Major League baseball. Just how bad is the situation for Los Angeles!

By patrick honner, 14 years8 years ago

Challenge Geometry Teaching

One-Cut Challenge: Quadrilaterals

Here’s another introduction to the one-cut challenge, this time with quadrilaterals. This is another activity for students of all ages from my Fun with Folding series, and you can try this out even if you haven’t fully explored the one-cut challenge with triangles.

Recall that in the one-cut challenge, your task is to produce the given shape as a cut-out using only straight folds and asingle straight cut.

Let’s start with a square. One simple approach is to fold the square in half, then fold the top and bottom halves up along the vertical side.

This same approach also works with rectangles.

A second approach with the square involves fewer folds. First, fold along the main diagonal, then go from there.

In addition to being a more efficient solution, and raising the general question “What is the minimum number of folds needed to produce the shape?”, this approach produces some confusing results when applied to a rectangle!