This video is a superb lesson on the inherent mathematics of tennis from the New York Times:
In breaking down a single return selection–down the line or cross-court–you get the flavor of how geometry, physics, probability, and game-theory all factor in to this instantaneous decision.
These folks at the New York Times really do an excellent job producing these videos.
A boat-builder once described to me one procedure for making oars.
Start with a long piece of lumber with a square cross-section. Take off a certain amount from each of the four corners. Now their are eight corners. From each of the eight corners, take off a smaller amount. The progression of cross-sections looks something like this:
Through practice, the builder knows how much wood to remove at each stage. You can continue to repeat this process, but some sanding at this point will probably get you pretty close to what you are looking for: a circular cross-section.
This naturally brings to mind the hallmarks of Calculus: approximations and limits. At each stage of the process, the cross-section of the oar becomes a better and better approximation of a circle. Indeed, the limit of such a process is indistinguishable from a circle.
I’m not sure if anyone making oars is thinking about Calculus, but sometimes it’s hard for me not to think about it!
Whenever I spend a lot of time driving, navigating, and map-reading, I find myself making a lot of rough estimates of distances. The process reminds me of how one estimates and ultimately evaluates, using Calculus, the arclength of a curve.
To find the length of a curve, we approximate the curve with a series of line segments. It’s easy to find the length of a line segment, and so by sacrificing exactness, you turn a hard problem into an easy one. This is a fundamental technique in Calculus.
I made my line segments equal in length to 40 miles on the map. Now just add up the lengths of the line segments to approximate the length of the curve.
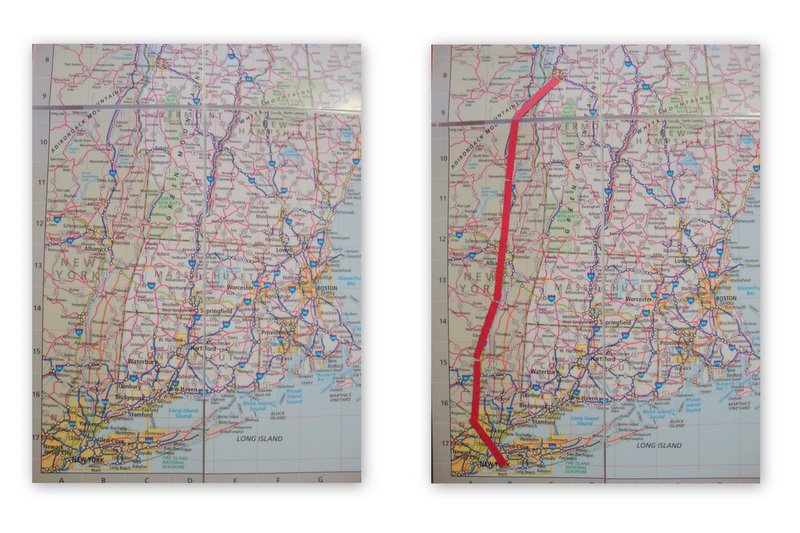
Each of the seven line segments is (roughly) equal to 40 miles, so the approximate length of the path from Brooklyn to Burlington is 280 miles (not a terrible estimate).
There are plenty of ways to improve the approximation, and the straightforward, but complicated, calculus approach eventually produces the arclength integral.
On the actual drive my approximations weren’t as good, as I was using an inferior distance estimator.
This is an absolutely mind-blowing idea: robotic “paper” that can fold itself into an arbitrary three dimensional object. Be sure to watch the short video accompanying the article.
http://web.mit.edu/newsoffice/2010/programmable-matter-0805.html
Tying (or folding?) all of the physics and engineering together here is the mathematics of origami. How can you fold a square sheet into a boat? A plane? A tetrahedron? A super-intelligent robotic giraffe?
I’ve seen better tricks, and setting up and executing this trick is no simple task, but there is definitely some cool mathematics behind this.
http://www.telegraph.co.uk/science/7950022/The-magic-of-mathematics-amaze-your-friends.html