Regents Recap — January 2018: Isn’t this Algebra?
What constitutes an algebraic solution? Let’s find out.
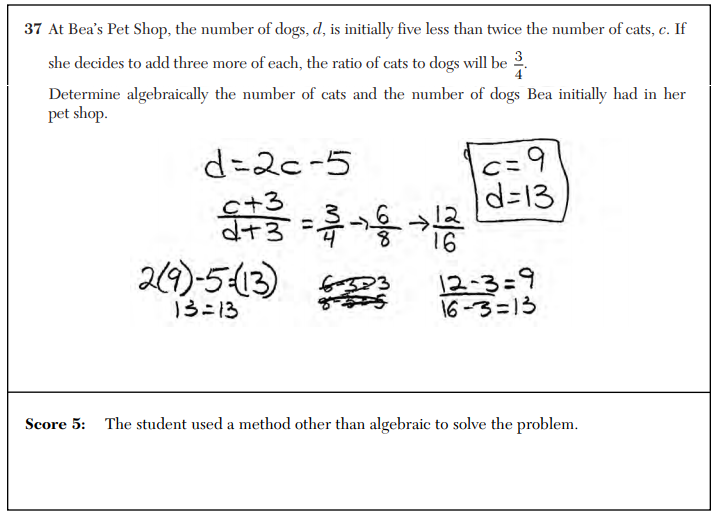
Here’s number 37 from the January, 2018 Common Core Algebra Regents exam. Only the final part of this six-point question is presented: Determine algebraically the number of cats and the number of dogs Bea initially had in her pet shop.
The key phrase here, apparently, is determine algebraically. If you don’t determine the answer algebraically, you can’t receive full credit. Here’s an example from the official Model Response Set in which the student loses a point for using “a method other than algebraic to solve the problem”.
In this solution, the student manipulates multiple equations, proportions, and equivalencies, determines the values of the variables that made the equations simultaneously true, and then applies substitution to verify their correct answer. That sure sounds like an algebraic solution to me.
Apparently it wasn’t the algebraic solution the test makers were looking for. But so what? We should be rewarding students for applying tools and techniques flexibly, not penalizing them for failing to adhere to a narrow, and secret, definition of what mathematics is.
Related Posts
- Regents Recaps
- Yes, You Can Work On Both Sides of an Identity
- Algebra is Hard
- How Do You Explain That 2 + 3 = 5?

4 Comments
Donny Brusca · February 22, 2018 at 10:29 am
I guess the issue they have is that an algebraic solution should not include any trial and error. The student tries various fractions equivalent to 3/4 until s/he hits upon one that works. (If 9/12 was the correct fraction, s/he would have missed it!)
I think what is worse is the part of this question that immediately precedes this:
Could Bea’s Pet Shop initially have 15 cats and 20 dogs? Explain your reasoning.
My answer would be “No, because in the next part of this problem I show algebraically that the Pet Shop initially had 9 cats and 13 dogs, not 15 cats and 20 dogs.”
How many points would I have gotten for that answer?
Donny
MrHonner · February 22, 2018 at 11:07 am
Donny-
The standard certainly can’t be “Can’t include any trial and error.” Testing out values can’t possibly disqualify an entire solution.
And as far as I’m concerned, strategic guessing-and-checking is a legitimate problem solving strategy, but to be honest I don’t see it here: I see a student deliberately manipulating a ratio into a form that makes the required algebraic relationship transparent.
And you raise a good question about the previous parts of the problem. I would give that answer full credit!
Donny Brusca · February 22, 2018 at 12:11 pm
OK, agreed. The use of trial and error (or guess and check) shouldn’t disqualify the solution. But I believe that what is meant by an “algebraic solution” is that the solution should not depend on these methods. We’d want to make sure the student can solve the same problem even if the correct fractional equivalent of 3/4 turned out to be 1095/1460 (ie, not something they can likely guess).
Long before common core, Regents required “algebraic solutions only” on certain problems, and in these cases, a trial and error method would not have received full credit. This is not new.
For example, here’s a question from the August 1987 Regents (Ninth Year Mathematics):
35. Find 3 consecutive positive integers such that the product of the first and third integers is 48. [Only an algebraic solution will be accepted.]
The student could guess the solution (6, 7, and 8) easily enough without any algebra, but would not have received full credit.
MrHonner · February 22, 2018 at 12:36 pm
I know about the scoring issue with trial-and-error. In fact, I recall that in order to get maximum credit for “trial and error”, you had to show at least three trials, even if your first guess was correct!
And I understand the point you are making, but as I said above, I don’t concede that this student is merely engaged in trial-and-error. I see legitimate algebraic work here.
The question of whether or not a test question like this should require the student to employ a specific technique (solving a system of equations using substitution, for example) is worthy of discussion. On occasion I’ll restrict students to specific strategies on a quiz or a test question, but generally I’m most interested in seeing if they can solve a problem or not, not execute a specific strategy. I tend to feel that this is how the Regent’s exam should be, as well.