Is 2+3i an Imaginary Number?
For over 10 years I have been writing and speaking about erroneous math test questions and their consequences. Question 25 from the June 2022 New York State Algebra 2 exam offers a clear and simple picture of those consequences.
The student is asked if the equation has “imaginary solutions”, that is, if the solutions to this equation, 2 +3i and 2 – 3i, are imaginary numbers. These solutions are complex but not imaginary, because imaginary numbers are multiples of i, the imaginary unit. Therefore the answer should be no, this equation does not have imaginary solutions.
As you might have guessed, that’s not the answer they were looking for.
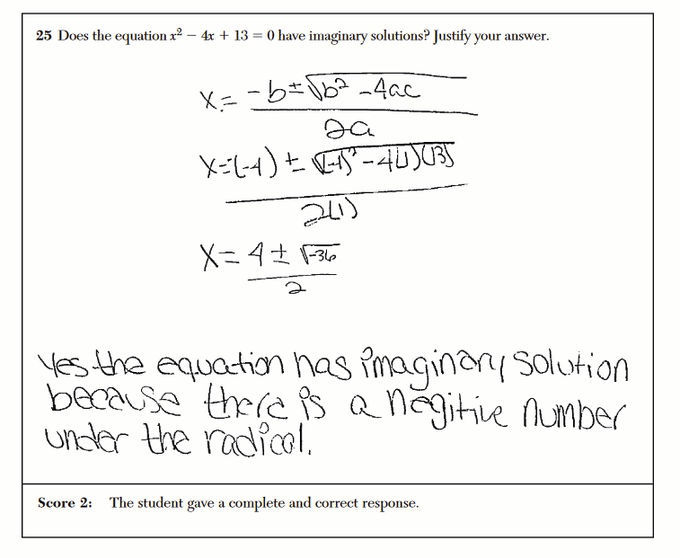
In this “complete and correct” response from the state’s official model response set, the student identifies these solutions as imaginary. These numbers are not real, but they are not imaginary, a subtle but meaningful distinction that neither the student nor the exam creators seem to understand.
Is the distinction important? Maybe not. But what is important is that this student’s lack of understanding of complex numbers will only be amplified by this exam. Even worse, teachers around the state might themselves be confused after reading this model response set. What will they teach their students about imaginary numbers next year?
Worst of all, what about the students who actually do know the difference between imaginary numbers and non-real complex numbers? They’re caught in a trap: Should they give the correct answer and possibly lose points, or should they try to guess what the exam creators really meant to ask? These tests put students in this trap over and over and over again, and ultimately students learn that details don’t matter and that thinking too much is a hazard. Students, and their teachers, deserve better.
Related Posts
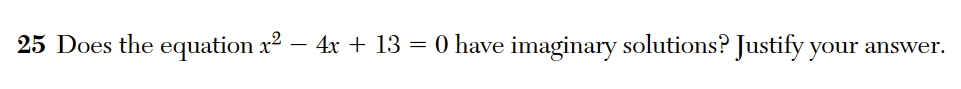
0 Comments