Seatings and Sums of Squares
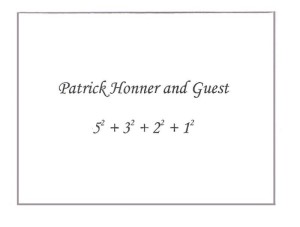 At Math for America events, guests are traditionally invited to find their seats in some math-y way. (This is just one of the many ways MfA uses math creatively at their functions). Here is my seating card from a recent dinner at the MoMA.
At Math for America events, guests are traditionally invited to find their seats in some math-y way. (This is just one of the many ways MfA uses math creatively at their functions). Here is my seating card from a recent dinner at the MoMA.
The keynote speaker, Dr. Eric Lander, is, among many other things, a mathematician-turned biologist who has been working on the human genome project. Dr. Lander gave a remarkably clear explanation of the inherent mathematics of genetics. And, as a lover of number theory, Dr Lander expressed some appreciation for the seating assignments.
Dr. Lander pointed out that the mathematical fact on display here is that every integer can be expressed as a sum of four squares. This is commonly known as the Lagrange Four Square Theorem.
Here are a few examples of the phenomenon:
Dr. Lander, a former International Mathematics Olympiad competitor, said that it is fairly easy to show that you need at least four squares to express every number as such a sum, but it’s much harder to show that you need at most four squares to get the job done.
Of course, the words “easy” and “hard” probably have unique meaning to someone cracking the human genome for a living!
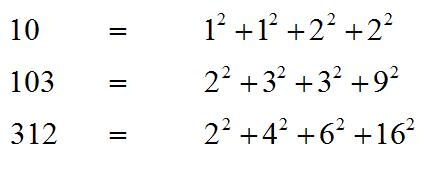
1 Comment
Ahmed Gouda · November 18, 2010 at 6:20 pm
Reminds me of proving any number in base 10 can be written in any base [insert positive integer] form. I question whether if base [insert positive fraction] could pull this off. Probably would lead to just writing up summations instead, then getting distracted by an interesting summation.