The other day in class during an exploration into solid geometry, we discovered we could make these lovely flowers
by smashing the paper cones we had made.
Now, if I can just figure out how to test the students on this material.
Related Posts
The other day in class during an exploration into solid geometry, we discovered we could make these lovely flowers
by smashing the paper cones we had made.
Now, if I can just figure out how to test the students on this material.
Related Posts
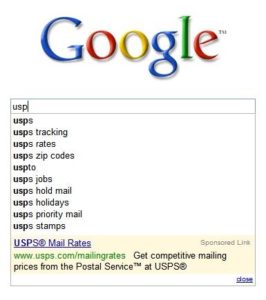 Through Math for America, I am part of an on-going collaboration with the New York Times Learning Network. My latest contribution, a Test Yourself quiz-question, can be found here:
Through Math for America, I am part of an on-going collaboration with the New York Times Learning Network. My latest contribution, a Test Yourself quiz-question, can be found here:
http://learning.blogs.nytimes.com/2011/03/16/test-yourself-math-march-16-2011/
This question is based on Google-search statistics, specifically how much traffic is driven to the top two Google search results.
Based on the numbers, it’s easy to see why companies are willing to bend the rules to climb the rankings!
 My latest contribution to the NYT Learning Network is a mathematics lesson build around the way NCAA basketball teams are ranked.
My latest contribution to the NYT Learning Network is a mathematics lesson build around the way NCAA basketball teams are ranked.
http://learning.blogs.nytimes.com/2011/03/14/whos-no-1e-investigating-the-mathematics-of-rankings/
Quantitative rankings are ubiquitous these days, and they are playing an ever-increasing role in teaching. Colleges have been “ranked” by publications for years, but now public schools are being assigned grades and even teachers can be ranked according to complicated, and often controversial, formulas.
In this lesson, students are tasked with creating their own rankings of the sports teams.
Looking only at winning percentage, therefore, may not be a fair assessment of who is better than whom: if Team X plays in a relatively weak conference, and Team Y plays in a relatively strong conference, it will be easier for Team X to record wins. This is similar to the idea of ranking students based on unweighted G.P.A.’s: such a system may well reward students who take relatively easy classes and put students with more challenging programs at a disadvantage.
So, student pairs should explore approaches to addressing this issue by attempting to quantify a team’s strength of schedule, thereby creating a ranking system that will take into consideration the quality of each team’s opponents.
These rankings only make as much sense as the underlying mathematics. Hopefully, through investigating the way the NCAA ranks basketball teams, teachers and students can learn to deconstruct these ranking systems and better evaluate their utility .
I admit it. The only reason I bought this squash
was to see if I could use Geogebra to fit a curve to it, like this.
And let me tell you, figuring out that this equation did the job
was not as easy as I thought it would be.
Related Posts