Teaching

Challenge Teaching

Fun With Folding: Basic Folds

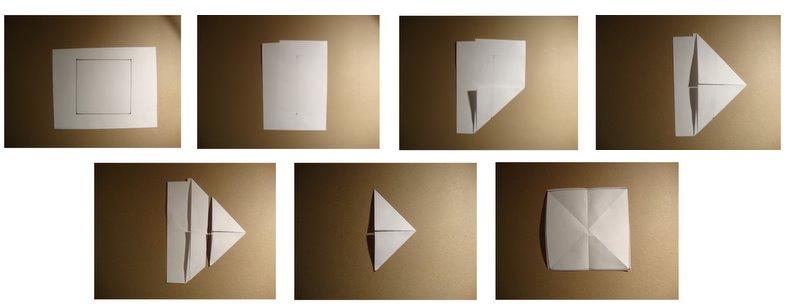
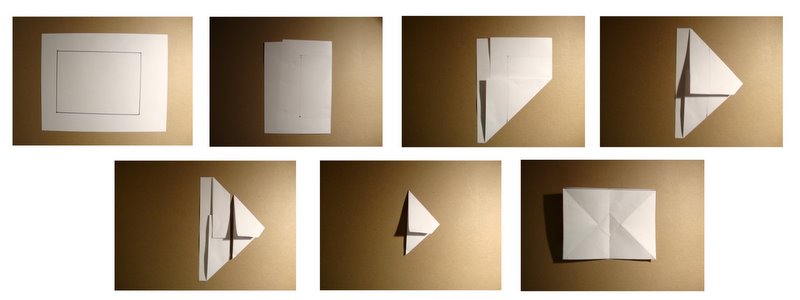
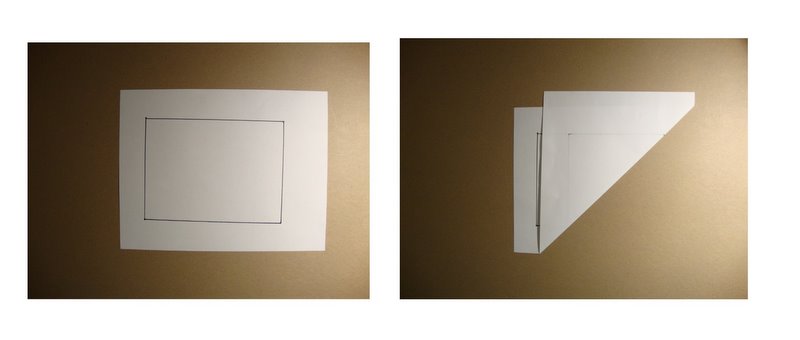
Here’s a good place to start my Fun With Folding series. Below are the instructions for four basic folds: folding a linethrough two points; folding the midpoint of a line segment; folding the perpendicular bisector of a segment; and folding the angle bisector of an angle. Get these all down and you’ll be ready to have lots of fun with folding!

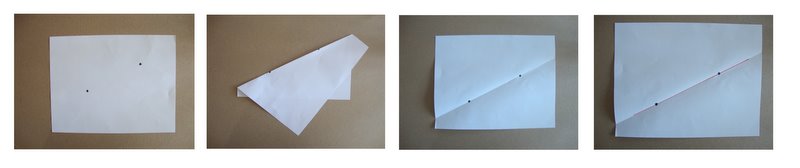
First, let’s fold a line between two points. The key here is to make sure that both points lie in the crease of the fold.

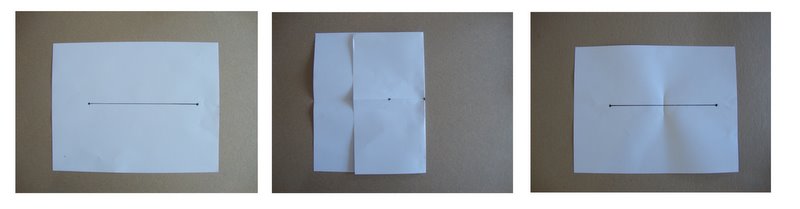
You can fold the midpoint of a line segment by folding in such a way that one endpoint of the line segment lies on top of the other. The two halves of the line segment should be right on top of each other, and just pinch the fold at the midpoint.

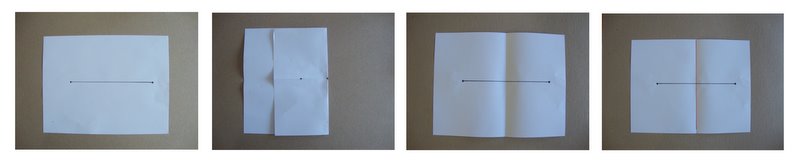
To fold the perpendicular bisector of a segment, perform the same steps as above for a midpoint, but just complete the crease!

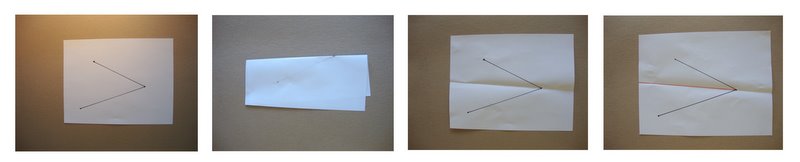
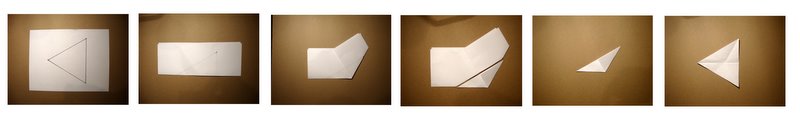
The last basic fold is an angle bisector. Given an angle, make a fold that passes through the vertex of the angle so that the two sides of the angle lie on top of each other.

Once you’ve got these basic folds down, you’ve got everything you need to try some more advanced folding challenges!

Have more Fun With Folding!

By patrick honner, 14 years6 years ago

Challenge Geometry Teaching

One-Cut Challenge: Quadrilaterals

Here’s another introduction to the one-cut challenge, this time with quadrilaterals. This is another activity for students of all ages from my Fun with Folding series, and you can try this out even if you haven’t fully explored the one-cut challenge with triangles.

Recall that in the one-cut challenge, your task is to produce the given shape as a cut-out using only straight folds and asingle straight cut.

Let’s start with a square. One simple approach is to fold the square in half, then fold the top and bottom halves up along the vertical side.

This same approach also works with rectangles.

A second approach with the square involves fewer folds. First, fold along the main diagonal, then go from there.

In addition to being a more efficient solution, and raising the general question “What is the minimum number of folds needed to produce the shape?”, this approach produces some confusing results when applied to a rectangle!

Another easy quadrilateral to work with is the isosceles trapezoid.

But watch out: an arbitrary quadrilateral poses quite a challenge!

Happy folding!

Have more Fun With Folding!

By patrick honner, 14 years6 years ago

Challenge Geometry Resources Teaching

One-Cut Challenge: Triangles

Here’s an introduction to the one-cut challenge using triangles, from my Fun with Folding series, suitable for students of all ages (including teachers!). This is a rich, compelling problem that touches on a lot of sophisticated ideas in geometry, but is simple enough to start playing around with right away.

The one-cut challenge is as follows: given a shape made up of connected straight line segments (i.e. a polygonal chain), can you produce the shape as a cut-out using only straight folds and a single straight cut?

A good place to start is with an equilateral triangle. This is a fairly easy problem to solve, given the inherent symmetry in the figure. Fold across any line of symmetry to produce a new figure that looks like two line segments meeting at an angle. Fold those together along their vertex, and cut!

The next step is trying this with an isosceles triangle, whose single line of symmetry still allows this approach to work.

Now the kicker: try it out on a scalene triangle! No more lines of symmetry, and all of the sudden this is a pretty challenging problem!

Happy folding!

Have more Fun With Folding!

By patrick honner, 14 years6 years ago

Geometry Teaching

A New Solution to an Old Problem

This story makes me feel bad for every time I discouraged a student from a math research project because the topic was too well-known.

http://novinite.com/view_news.php?id=122377

A 19-year old Bulgarian student has solved the 2000-year old Problem of Appollonius in a new and unique way. It is the first new solution in 200 years, and only the fifth known solution overall.

The Problem of Appolonius, essentially, is to construct (with straightedge and compass, only) a circle that is tangent to three given objects. Here is an example of an Appolonius Circle (in red) that has been constructed to be tangent to the three given circles (in black).

This story is nice reminder that sometimes the best thing to do as a teacher is get out of the student’s way!

By patrick honner, 14 years8 years ago

Appreciation Resources Student Work Teaching Technology

Student Work: Curvefitting With Geogebra

Here is some student work from a recent project I conducted on fitting curves to images in Geogebra. The details of the assignment can be found here, and more examples of student work can be seen on my Facebook page.

Students were asked to find pictures and use Geogebra to fit trigonometric curves to the images using transformations. Here are some of the results.

Smart Water = Smart Curves

My Good-Looking Windowsill

Sine of Camel Humps

Overall, I was really impressed with the creativity the students showed, and their facility with fitting these curves to the forms! A mathematical and artistic success in my book.

Follow Mr Honner