Probability

Are all of us descendants of Confucius?  Here’s a curious mathematical argument that suggests just that.

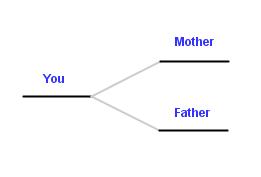
No matter who you are, you came from a mother and a father (I won’t go into details).  So, in your family tree, the part behind you has two branches, like this:

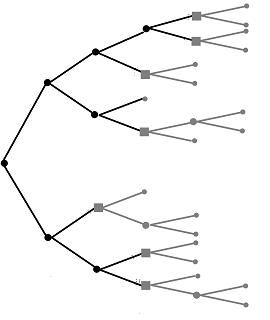
The same goes for your mother and father, and their mothers and fathers, and so on.  Thus, continuing on back the line, you see a family tree like this

And it just keeps going and going and going.  An interesting mathematical feature of this tree is that, as your move backward in time, each generation has twice as many branches as the previous generation, roughly speaking.  Thus, when you go back a hundred or so generations, to the time of Confucius, the number of branches in your family tree is roughly , or 633,825,300,114,114,700,748,351,602,688 (thanks, WolframAlpha).

A reasonable estimate is that at the time of Confucius there were around 250 million total people in existence.  Each of those spots in your family tree has to be filled by someone, which means that, on average, each person in existence at that time had to fill roughly

=  2,535,301,200,456,458,802,993

of the spots in your family tree.   It seems like a statistical impossibility that Confucius wasn’t one of them.  So, I guess that makes us cousins?

I just donated this shirt to the Salvation Army.  I estimate the probability that I will ever see this shirt again to be zero.  In fact, forget estimating:  I think the probability that I will see this shirt again is exactly zero.

In fact, I’ll go even further than that:  I claim that the probability that anyone who reads this ever sees this shirt is exactly zero.  Not .0000000000001.  Zero.

Even if there are infinitely many possible scenarios in which this shirt is seen by someone, this number is dwarfed by the infinitude of possible scenarios in total.  Thus

moderate infinity  ÷  really big infinity = zero

I dropped it off at the Salvation Army in Brooklyn.  Let me know if you see it:  evidence will be required to claim your prize.

For the second consecutive day, I unexpectedly crossed paths with an acquaintance.  In both cases, the encounter occured outside my daily routines, the person was someone I know only minimally, and we were both en route to different destinations when we happened to notice each other.

There are a lot of interesting results associated with objects moving around randomly in a given space, but as is usually the case with mathematics, the situations are idealized to eliminate some of the complicating real-world issues.  Imagine a dot moving back and forth on the number line, or randomly around the Cartesian plane, for example.

I’m not really sure how unlikely it is for me to have two serendipitous encounters on back-to-back days, but the following questions are probably significant:  how dense is the population?  How many people do I know?  How attentive am I?  How popular is my destination?  There are probably many more other important and complicating factors.

In any event, it’s certainly unlikely that it will happen again today.