Appreciation

Appreciation Art Geometry Resources Student Work Teaching

Fun With Sliceforms

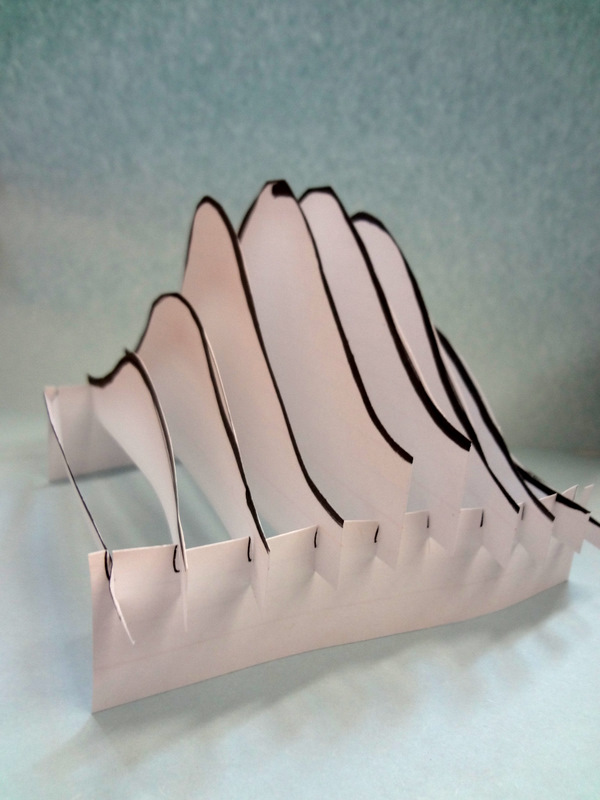
I was recently inspired to make my first sliceform.

With a handful of index cards, a marker, and some scissors, I was able to make this fun representation of a surface in 3D!

Turn it to the side, and see the surface from a different perspective.

The inspiration was timely, as my Calculus class has been discussing cross-sections, traces, and level curves of surfaces in space. What a perfect way to demonstrate how to understand a surface by looking at representative slices!

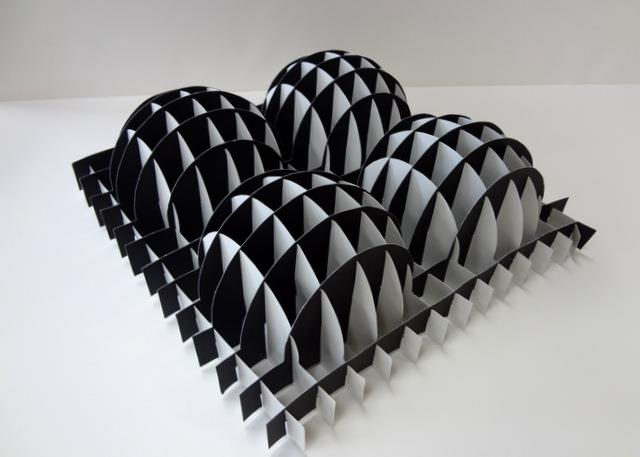
A great, simple tool, and you can see some examples of the sliceforms my students created, like the one seen below, here.

By patrick honner, 13 years9 years ago

Appreciation Art Photography

Math Photo: Triangular Trim

This is a nice way to decorate the side of a building.

By patrick honner, 13 years4 years ago

Appreciation Resources

Mathematical Quotations

This is a fun collection of mathematical quotations compiled by a professor at Trent University:

http://euclid.trentu.ca/math/sb/misc/quotes.html

The list includes some classics, such as the following famous remark from John von Neumann:

“In mathematics, you never understand things; you just get used to them.“

I also like this quote, attributed to Hungarian mathematician Raoul Bott:

“There are two ways to do great mathematics. The first way is to be smarter than everybody else. The second way is to be stupider than everybody else — but persistent.”

To add a favorite recent quote of mine to the list, a teacher was discussing how she used the idea of friendly numbers to help her quickly calculate in her head. But while explaining the process, she warned us to be careful: “Remember, what’s friendly to me may not be friendly to you!“.

By patrick honner, 13 years4 years ago

Appreciation History

Obvious, but Difficult

This is a fun conversation on MathOverlflow.net about famous examples of theorems in mathematics that are “obvious” but very difficult to prove.

http://mathoverflow.net/questions/51531/theorems-that-are-obvious-but-hard-to-prove

For example, the Jordan Curve Theorem essentially states that any closed curve in the plane divides the plane into an “inside” and an “outside”. Obvious, right? But very difficult to prove.

The Isoperimetric Theorem is another good example. This theorem basically says that the most efficient way to surround area in the plane is with a circle. Again, easier to believe than to prove.

And one of the responders notes that, after taking several hundred pages in their Principia Mathematica to prove that $1 + 1 = 2$ , Russell and Whitehead note that the proposition “is occasionally useful”.

“Obvious” is one of the most dangerous words in mathematics!

By patrick honner, 13 years4 years ago

Appreciation Photography

Math Art: Weaving the Plane

While at the 2011 Bridges Conference, I learned a bit about the mathematics of weaving. One of our projects was to plan and weave a tiling of the plane. For some unknown reason, I chose a Halloween theme.

By patrick honner, 13 years4 years ago

Follow Mr Honner