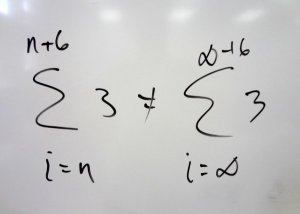As previously noted, there is plenty of mathematical fun to be had at Ikea!
And if taking pictures of cool tilings gets dull, why not trying tiling the plane yourself!
Appreciation Teaching
Scenes from Math Class: An Elegant Proof
I enjoy making students draw diagrams like these in their notes. You’ll never understand how difficult it is to draw mutually tangent circles until you try to do it yourself!
For more images from math class, check out my Facebook page.
Click here to see more in Teaching.
Appreciation Teaching
Scenes from Math Class: Infinite Sums
We always end up arguing about stuff like this in my class.
For more images from math class, check out my Facebook page.
Application Appreciation
Rent Stability
As usual, I wasn’t thrilled when my landlord told me about this year’s rent increase. As someone familiar with how exponential growth works, I understand the power of the small, consistent percent increase.
After some mild protests, the landlord came back with some good news: he was going to reduce my increase by $25 per month! I wasn’t exactly thrilled with that, either, but it’s a gesture. And I thought to myself “At least I won’t be paying future increases on that $25!”
Which got me thinking: if my rent goes up 3% every year, how much of a difference will that $25 make? The answer: not much. In my lifetime, anyway.
At 3% per year, the $25 difference in rent now will be a $30 difference in 5 years. In 15 years, the difference in the two rents will be around $40. And if I stick around for 50 years, the difference will be about $90.
If I could stay here for 200 years, I’d see a big difference: almost $10,000! But then again, my rent would be around $1 million per month.
I’ll have to be content knowing that, while in the short term I don’t see much benefit, I win out as t goes to infinity.
Appreciation Geometry
This is Not a Rectangle
After having fun exploring rigid and non-rigid frames, I hung one of our indeterminate quadrilaterals up on the board. The next day, we were proving a theorem about orthodiagonal quadrilaterals, and the final step concluded that a particular quadrilateral was actually a rectangle.
I found a cute little spot to finish our proof.
This elicited a few laughs from students who appreciated the irony.
But apparently, some students in a later class did not appreciate it. They felt the need to chime in.
As a general rule I must oppose mathematical graffiti, but it’s hard not to respect their position.