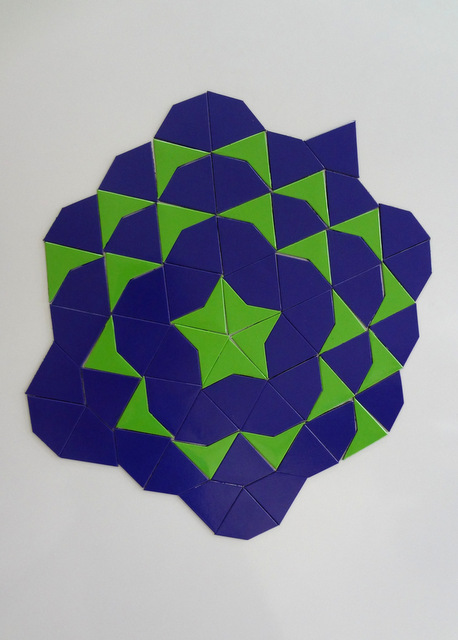
I’m greatly enjoying my Penrose Magnets. And I’m following the rules this time!
Appreciation Challenge Student Work
Custom Pi Necklace
In honor of Pi Day, here’s a wonderful example of student creativity: a necklace that encodes the first 80 or so digits of pi in beads!
Starting with the pendant as 3, the beads and string clockwise, in a circle, according to the following mapping:
Thus, you can read off 3.14159265358, and so on. A truly thoughtful, creative, and inspired work!
Related Posts
Appreciation Resources
Penrose Magnets
Well, it took a while, but my Penrose Magnets have finally arrived! We are going to have a lot of fun with these.
Appreciation Art Photography
Math Photo: Unfolding Symmetry
As this lovely purple flower opens and expands, it suggests a rotational symmetry about its center.
Appreciation Numbers
How Many Primes Did We Miss?
The mathematics world is abuzz with the verification of a new largest known prime number. The number, 
It’s interesting to note that, while it has been known for thousands of years that there are infinitely many primes, it is a challenge even today to find large ones.
It is also interesting to note how many primes were missed in jumping from the previous largest prime to this new largest prime.
A well-known and elegant result, Bertrand’s Postulate, states that there is always a prime number between n and 2n, for n > 1. For example, the prime 3 is between 2 and 4; the prime 5 is between 3 and 6; the prime 11 is between 10 and 20; and so on.
In particular, this says that there must be a prime between 
Thus, there must be a prime between 
Thus, there are at least 57,885,161 – 43,112,609 = 14,772,552 primes between 
Let’s hope it’s not another 4 years until we have a new largest prime on the block.