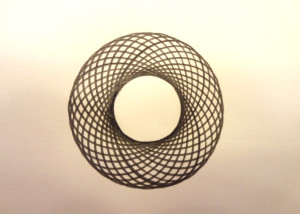
This hypotrochoid art set was the perfect stocking stuffer for a math lover like myself! With a little practice you can quickly produce some really beautiful images.
Appreciation Geometry Uncategorized
Fibonacci Flushers
While travelling in Europe, I became fascinated with the variety of toilet-flushing mechanisms I encountered. The typical toilet had a low-flow / high-flow option (which I imagine saves a great deal of water in the long run) , and a lot of creativity emerged in the way this two-flush system was implemented.
While documenting the many ways to flush, I found this rectangular model oddly familiar and appealing.
And then it hit me: this looks like the golden rectangle!
The golden ratio has long been used by artists and architects to create aesthetically pleasing work. It is, after all, the divine proportion. Could it be that these toilet-makers took their cues from the masters of art and math? I had to find out.
I dropped my image into Geogebra and took some measurements.
The total length of the rectangle divided by its height is around 1.71. So, it’s not quite the golden ratio, but it’s pretty close. This flush-design is about 90% divine, I suppose.
Maybe their next design will be closer to the perfect proportion.
Appreciation Photography
Math Photo: Urban Buckyball
There’s something a bit spooky about this sculpture of nested, truncated icosahedra in Madson Square Park. The eerie lighting and coloring brings to mind an evil, robotic Jack-o-Lantern.
Related Posts
Appreciation Geometry
IKEA and the Packing Problem
You know who is really good at geometry? IKEA.
The efficient assemblage of triangles, rectangles, and irregular pentagons is impressive enough, but check out this clever use of an empty quarter circle!
An appropriately sized shim lets a couple of rectangles fit perfectly into that corner!
Appreciation Geometry
Math Photo: Playground Polyhedron
I like how this piece of playground equipment is designed to demonstrate some mathematics: the braces are color-coded by degree. For example, the blue braces are vertices of degree 6; the red braces are vertices of degree 5; and the green braces are vertices of degree 4.
The color-coding makes it easy to see the overlap of polygons here, too: around each red vertex is a pentagon, and around each blue vertex is a hexagon.
I’m pretty sure this is a bit of cheat as far as regularity goes, but it’s still a nice piece to play with!