MrHonner

Geometry Teaching

The Closest Point on a Parabola to a Point on its Axis

While playing around with distances from points to graphs, I discovered the following interesting property of parabolas.

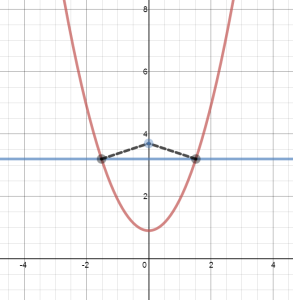
Suppose you have a standard parabola, $y = x^2$ , and a point on its axis of symmetry, $(0,a)$ . Then the point on the graph of the parabola closest to the point $(0,a)$ either has y-coordinate equal to $a - \frac{1}{2}$ , or is the vertex of the parabola.

A calculus-based proof is fairly straightforward.

The distance between a point on the parabola, say $(x, x^2)$ and the point $(0,a)$ is given by

$d(x) = \sqrt{x^2 + (x^2-a)^2}$

To find the minimum distance, differentiate $d(x)$ to get

$d'(x) = \frac{2x + 2(x^2-a)2x}{2\sqrt{x^2 + (x^2-a)^2}}$

This derivative is undefined when the denominator is zero, which only happens when the point $(0,a)$ is the vertex. The derivative is zero when

$2x + 2(x^2-a)2x = 2x ( 1 + 2(x^2 - a)) = 2x(1 + 2x^2-2a)=0$

By the zero-product property, either $x = 0$ or $1 + 2x^2-2a = 0$ .

The critical value $x = 0$ corresponds to the vertex of the parabola. The distance from $(0,a)$ to the vertex is a minimum if $a \leq \frac{1}{2}$ , or a relative maximum when $a > \frac{1}{2}$ .

Now, if $1 + 2x^2 - 2a = 0$ , we have $x^2 = a - \frac{1}{2}$ . Since $y = x^2$ on the parabola, the point associated with this critical value has y-coordiante $a - \frac{1}{2}$ . Some simple analysis shows that the distance to this point is minimal.

As an alternative to optimization, one could approach the problem geometrically. Since the shortest path from a point to a line is the perpendicular line segment connecting them, the shortest path between a point and a curve should be a line segment perpendicular to a tangent to the curve. At a point on the parabola $(x,x^2)$ , the slope of the tangent line is $2x$ , and so the slope of the line perpendicular to the tangent at $(x,x^2)$ would be $-\frac{1}{2x}$ . This is equivalent to $-\frac{\frac{1}{2}}{x}$ , and since $x$ is the distance to the axis of symmetry, it follows that this line intersects the axis of symmetry at a point a half-unit up in the y-direction.

This fun and surprising result would make a nice exploration in a Calculus class. It easily extends to general parabolas, and the situation naturally suggests some compelling extension questions.

You can explore the problem, and create variations, in this interactive Desmos demonstration I put together.

By MrHonner, 11 years11 years ago

Appreciation Geometry Photography

Math Photo: Flowery Projection

A timely combination of falling leaves and falling rain produced this lovely and unusual shadow.

This reminds me of taking the projections of curves and surfaces in space. Looking at an object’s shadows in various directions can often help you understand the nature of the object itself.

By MrHonner, 11 years11 years ago

Application NYT Resources Teaching

Exploring Fair Division

My latest piece for the New York Times Learning Network is a math lesson exploring basic techniques of fair division.

Fair division is concerned with partitioning a set into fair shares. “Fair” can take on different meanings in different contexts, but at its most basic level, a share is fair if someone is willing to accept it.

This lesson builds on an excellent article in the NYT about a technique in rent-splitting based on Sperner’s Lemma, an important result in Topology. The author tells the story of how he and two roommates used the technique to settle on a fair division of rent for three different-sized rooms.

“The problem is that individuals evaluate a room differently. I care a lot about natural light, but not everyone does. Is it worth not having a closet? Or one might care more about the shape of the room, or its proximity to the bathroom.

A division of rent based on square feet or any fixed list of elements can’t take every individual preference into account. And negotiation without a method may lead to conflict and resentment.”

After reflecting on the article, students use the related NYT interactive feature to explore the algorithm and then research basic techniques in fair division like divider-chooser, sealed bids, and the method of markers. The full lesson is freely available here.

By MrHonner, 11 years10 years ago

Appreciation Art

Math Art: Bird Rosetta Tiling

This lovely tiling by bird rosettas is the result of a yearly workshop for industrial design students at the Eindhoven University of Technology. You can read more about the work here.

This is another piece from the 2013 Bridges Mathematical Art Gallery.

By MrHonner, 11 years11 years ago

Challenge NYT

Math Quiz — NYT Learning Network

Through Math for America, I am part of an ongoing collaboration with the New York Times Learning Network. My latest contribution, a Test Yourself quiz-question, can be found here

Test Yourself — Math, May 14th, 2014

This question is about a Queen’s resident who made nearly $18,000 last year by renting out his spare room to visitors through the website Airbnb. Approximately how many nights did he have paying guests in his home?

By MrHonner, 11 years7 years ago

MrHonner

Follow Mr Honner