MrHonner

Regents Exam Recap: August 2012

Here is a brief review of the August 2012 New York State Math Regents exams, as part of my on-going series examining the quality and validity of these tests.

Only the Integrated Algebra and Geometry exams were offered in August 2012, presumably due to financial constraints.  Here are a few thoughts regarding some selected questions from those tests.

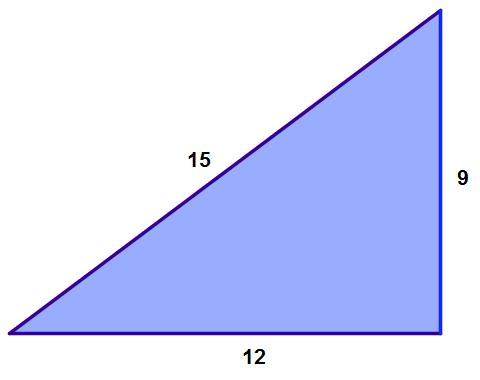
First, some issues that are probably minor to most, but important to me as a mathematician and a teacher.  Here is number 26 on the Geometry exam.

What does it mean to “use” a point in drawing a triangle?  Obviously, the author meant that the given points were to be the vertices of the triangle, but why not say that?  Technically, I could use a point by drawing a line segment through it; as such, I could draw any kind of triangle using the given points.

Number 3 on the Integrated Algebra exam includes a particular pet peeve of mine.

There is no value of y in the given equation.  In fact, that’s the entire point of a variable:  it can take any value!  What the author means is “What value of y makes the following equation true?” , so why isn’t that the question?  In addition to asking a question that actually has a correct answer, this would also model for students the correct way to think about variables and equations.

Here’s another problem from the Integrated Algebra exam, number 36:

There’s nothing mathematically wrong with this particular question.  However, the Integrated Algebra exam is the first of the high school mathematics exams in New York City.  It is meant to be taken after an introductory algebra course, which means that most students will take this test in 9th or 10th grade.

Thus, it is strange to note that this question, on the 9th grade exam, is virtually the same as the highest-valued question on last year’s Algebra 2 / Trigonometry exam.  The Algebra 2 / Trig exam is highest level math exam in the state.   It is usually taken in 11th or 12th grade.

So where should this question be?  Is it part of the 9th-grade curriculum?  Or the 11th-grade curriculum?  The authors of these exams seem a bit confused about this.

In addition, the August 2012 Geometry exam contained one of the most embarrassing math Regents questions I’ve ever seen, but I’ll address that in a separate post.

By MrHonner, ago

Derivatives of Even Functions

A recent tweet from @AnalysisFact noted that the derivative of an even function is an odd function.  There are many ways to explore and understand this fact, but here’s a simple algebraic approach that uses a neat little trick in representing even and odd functions.

Claim:  The derivative of a [differentiable] even function is an odd function.

Proof:  Suppose f(x) is an even, differentiable function.  Consider the function

a(x) = \frac {f(x) + f(-x)}{2}

First we show that a(x) = f(x).  Since f(x) is even, we know

f(x) = f(-x).

Thus, a(x) = \frac{f(x)+f(-x)}{2} = \frac{2f(x)}{2} = f(x).

Now let’s differentiate f(x).  We have

f'(x) = a'(x) = \frac{(f(x)+f(-x))'}{2} = \frac{f'(x) - f'(-x)}{2}

where the last step follows by the chain rule.

And since

f'(-x) = a'(-x) = \frac{f'(-x) -f'(x)}{2} = -\frac{(-f'(-x)+f'(x))}{2} = -a'(x) = -f'(x)

we see that the derivative of f(x) is an odd function, as desired.

Related Posts

 

By MrHonner, ago
Follow

Get every new post delivered to your Inbox

Join other followers: