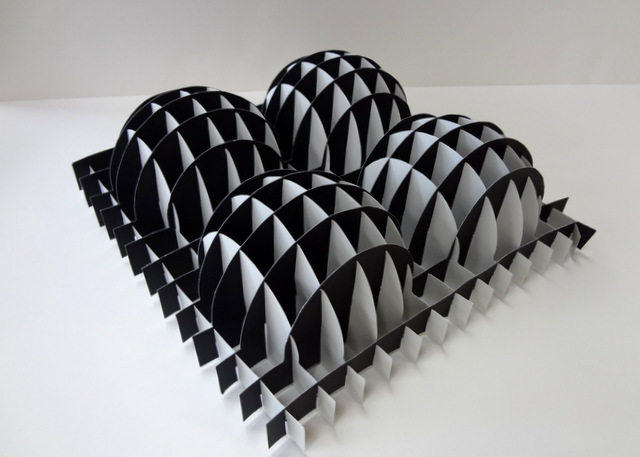
As a project in my Calculus course, students built sliceforms. Some of them turned out quite beautifully!
Sliceforms highlight the way we can use two-dimensional cross-sections to understand and represent surfaces in three dimensions.
You can see more examples of student sliceforms here, on my Facebook page.