Regents Recap — January 2018: Promoting Bad Habits
As an AP Calculus teacher, I looked upon number 30 on the January, 2018 Algebra 2 exam with great trepidation.
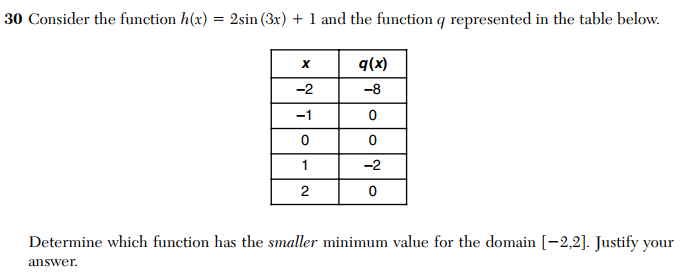
Functions are frequently given as tables in AP Calculus. This alternate representation of function helps create a numerical bridge between their formal and graphical representations, and it can also establish connections with data and statistics. If nothing else, the ability to represent and understand mathematical objects in different ways is incredibly powerful.
But it’s crucially important to understand that tables, by design, represent only a small sample of a function. Unless we have other information, there’s very little we can say about what the function does outside the table values. In particular, we have no reason to believe that the largest value in the table is the largest value the functions takes, either globally or locally: the function could do practically anything in between the given values. This is a common misconception among calculus students, and it takes consistent effort to correct it.
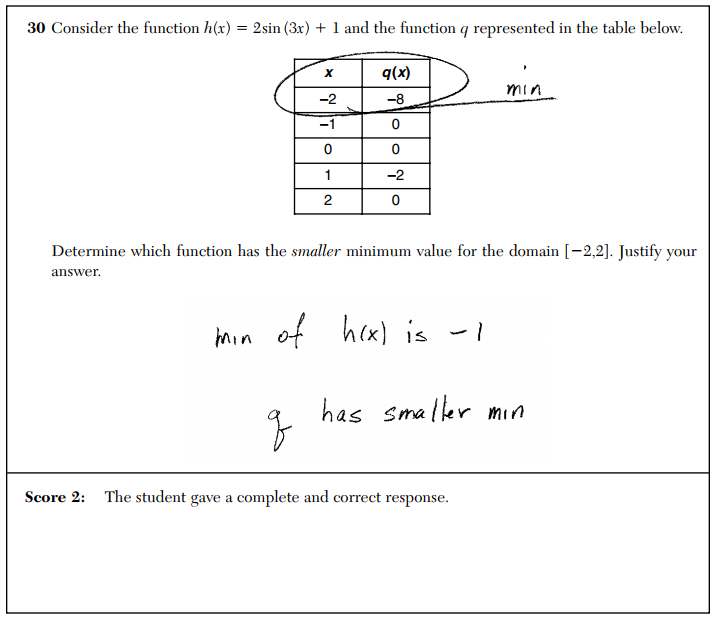
Thus, I was worried to see a Regents question asking about extreme values for a function given as a table. As it turns out, there’s nothing mathematically wrong with this particular question: the given trigonometric function is bounded between -1 and 3, so the fact that q(x) takes a value of -8 means it must take the smaller minimum value. However, it would be a mistake to claim that the minimum value of q(x) is -8, as the function could potentially drop below -8 between any pair of given values on that interval.
So it’s disheartening to see student work that makes this exact claim labelled as “complete and correct”.
I think this is a fairly reasonable answer for an Algebra 2 student. And I don’t entirely blame them for not fully understanding the subtleties of the correct argument: that the minimum value of q(x) must be less than or equal to -8, which is less than the minimum value of h(x), and therefore q(x) must have the smaller minimum value.
But I do blame the test makers for not fully understanding the subtleties of the correct argument. And I blame them for writing yet another test question that promotes bad mathematical habits, by expecting and rewarding an incomplete answer, and setting students up for deeper misconceptions later on down the line.
Related Posts
7 Comments
MrHonner · February 28, 2018 at 1:19 pm
On Twitter, mathematician Rachel Quinlan points out that the question’s assumption that q(x) must take a minimum value on [-2,2] is also pretty bad.
Anders Hulleberg · February 28, 2018 at 9:12 pm
I have two honest questions.
1) An incomplete sentence with no punctuation counts as a “fairly reasonable” answer?
2) What if the student had not circled the first entry in the table and labeled it a min? What if he/she had simply written the following? “The minimum of h is -1. Since q(-2) = -8, q must have a smaller minimum.” Would it still trouble you to count as complete and correct?
MrHonner · February 28, 2018 at 10:11 pm
I’m judging only the mathematical content of the response, and strictly in the context of how Regents exams are scored. (An incomplete sentence or a lack of punctuation would never result in a penalty on one of these math exams, for example.)
Your hypothetical response is clearly better, but my primary concerns here lie with the test makers, not the possible responses from test takers.
Anders Hulleberg · March 1, 2018 at 8:50 am
OK, thanks. So the issue here is that the student was rewarded for erroneously concluding that -8 must be the minimum of q. Got it.
Does Rachel Quinlan’s observation mean that the question is impossible to answer? If q has a minimum on [-2,2], then it must be less than -1. If q doesn’t have a minimum on [-2,2], then by default h has the smaller minimum. Either case is possible, and the question doesn’t indicate which case should be assumed, so the question can’t be answered definitively. Is that right?
MrHonner · March 1, 2018 at 12:29 pm
Rather than impossible, I would say that if q(x) doesn’t take a minimum value (which it need not do), then the question “Which function has the smaller minimum value?” is meaningless.
It would be weird to say that h(x) has the smaller minimum value if q(x) were unboundedly negative! Though I get your point.
Alax · March 10, 2018 at 3:19 pm
Shouldn’t q(x) be interpreted as only those five discrete points instead of as being continuous?
MrHonner · March 10, 2018 at 7:07 pm
The directive is to “Determine which function has the smaller minimum value for the domain [-2,2]”. This clearly implies that the domain of q(x) is [-2,2].
But it doesn’t imply it’s continuous, which isn’t a concept students in this course would be familiar with.