Happy 2016!
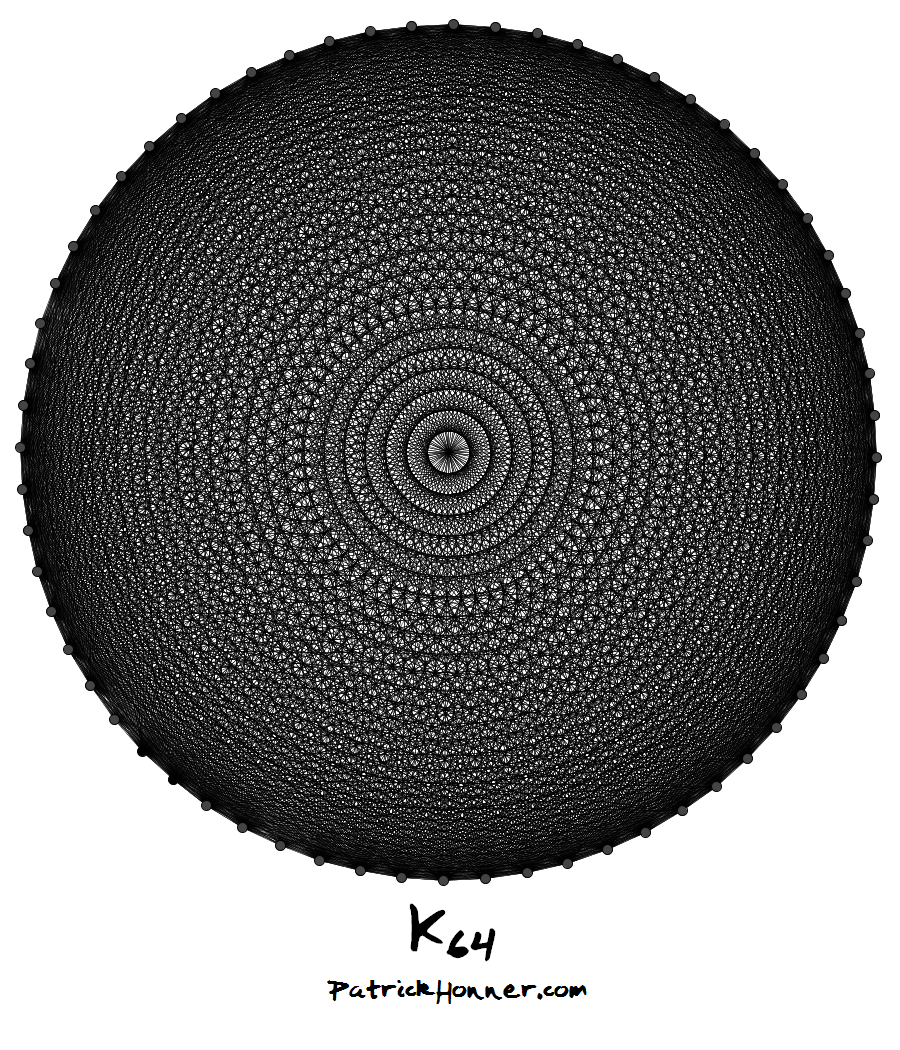
In honor of the new year, here’s the complete graph on 64 vertices, with its 2016 edges!
A complete graph is a graph in which every pair of vertices is connected with an edge. In a complete graph with n vertices, there are
edges. The above graph has 64 vertices equally spaced around the perimeter. Thus, , and we have
edges.
The number 2016 is special for a variety of reasons. For example,
So 2016 is equal to the sum of the first 63 positive integers! This makes 2016 a triangular number, a fact beautifully demonstrated by David Swart in this image.
And John D. Cook illustrates the combinatorial nature of 2016 by pointing out that this is the number of ways to place two pawns on a chessboard!
However you think of it, 2016 is a pretty great number! And here’s hoping 2016 is a great year.
2 Comments
Irene Espiritu · January 3, 2016 at 3:14 am
Cool. I think 2016 is also the numerical coefficient of the third term of the expansion of (a + b)^64= a^64 + 64 a^63 b + 2016 a^62 b^2 + …… A Happy and Prosperous 2016 for all of us!
Sheila Krilov · January 3, 2016 at 2:05 pm
Awesome!!!
What a mathematical start to the New Year!
PS Here’s an old classic updated:
A circle is inscribed in a right triangle so that the point of tangency divides the hypotenuse into two segments whose lengths have a product of 2016. Compute the area of the triangle. [thanks to Richard Kalman]