12/30/2013 — Happy Derangement Day!
Today we celebrate a Derangement Day! Usually I call days like today a permutation day because the digits of the day and month can be rearranged to form the year, but there’s something extra special about today’s date:
The numbers of the month and day are a derangement of the year: that is, they are a permutation of the digits of the year in which no digit remains in its original place!
Derangements pop up in some interesting places, and are connected to many rich mathematical ideas. The question “How many derangements of n objects are there?” is a fun and classic application of the principle of inclusion-exclusion. Derangements also figure in to some calculations of e and rook polynomials.
So enjoy Derangement Day! Today, it’s ok to be totally out of order.
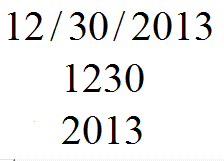
1 Comment
Alan Hochbaum · December 31, 2013 at 10:06 am
Don’t think this is right, but felt like I was getting warm? Tepid?
(n-1)[(n-1)!+(n-2)!]