Infinite Prime Gaps

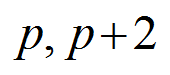The mathematics world is abuzz with news that someone may have proved a weak version of the Twin Prime conjecture.

A pair of numbers are called twin primes if the two numbers are both prime and they differ by 2.  Examples of twin primes include 11 and 13, 29 and 31, and 137 and 139.  Notice that for all prime numbers other than 2, twin primes are as close as two prime numbers could possibly be:  the number between the twin primes will always be even, and thus not prime.

The Twin Prime conjecture simply postulates that there are infinitely many pairs of twin primes.  Although it is simple to state, the Twin Prime conjecture has been hard to prove:  it has been an open question in Number Theory for hundreds of years.  But a breakthrough has been made.  Someone apparently has proved that there are infinitely many pairs of primes that differ by at most 70 million!

Now, being 70 million apart isn’t the same as being 2 apart, so at first glance this result may not seem significant or relevant.  But the difference between 70 million and 2 is nothing compared to the difference bewteen 70 million and infinity!  Essentially, this result says that no matter how far out you go on the number line, you can always find two primes that are relatively close to each other, where relatively close here means “no more than 70 million apart”.

And while being 70 million away may not seem close as far as prime numbers go, consider the following amazing fact:  given any number N, we can find a string of N consecutive numbers that contains no primes at all!  That is, we can find “gaps” between the primes as large as imaginable:  70 million, 700 million, 7 trillion trillion, and beyond.  What’s more, it’s quite easy to prove this fact.

Consider .  Since n! is the product of all the integers from 1 to n, it is clear that every integer less than or equal to n divides n!.

Now, since n! is divisible by 2, we know (n! + 2) must also be divisible by 2.  Similarly, since n! is divisible by 3, then (n! + 3) must be divisble by 3, and so on.   Thus, we have the following sequence of n-1 consecutive numbers

none of which are prime!  For example, if n = 5, the numbers 5! + 2, 5! + 3, 5! + 4, and 5! + 5 are

122, 123, 124, 125

which are are consecutive and not prime.

Using this technique, we can generate strings of consecutive non-primes of any length.  For example, if we let n = 70 million, we’ll get a string of 70 million – 1 consecutive non-primes.  Or if we let n = 1 googol (), we’ll get a string of   consecutive non-primes!

This technique shows if we go out very far on the number line we are sure to find huge gaps bewteen prime numbers.  But according to the new mathematical result, no matter how far out we go, we can always find primes that are relatively close to each other.

This is a major result, and an exciting day for mathematics!