I’ve seen better tricks, and setting up and executing this trick is no simple task, but there is definitely some cool mathematics behind this.
http://www.telegraph.co.uk/science/7950022/The-magic-of-mathematics-amaze-your-friends.html
Appreciation Geometry
Garden-Variety Fractals
My Mom gave me some clippings of a Christmas Cactus (Chris, pictured at the right), and after taking a while to get accustomed to her new home, Chris is finally starting to grow.
The new leaves sprouting out, smaller but similar to the original, put me in mind of the Mandelbrot set. The bulbs that “grow” out of the Mandelbrot set are perfectly similar to the original, and no matter how much you zoom in, you’ll always see the exact same sort of object.
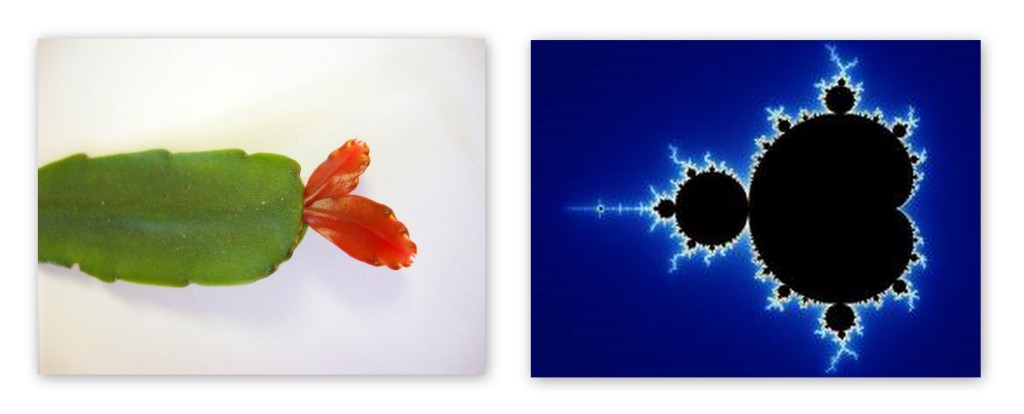
I can’t say for sure if the leaves of the Christmas Cactus are infinitely self-similar, but it’s close enough for my eyes.
Appreciation Game Theory
Game Theory and Rock-Paper-Scissors
This is an amusing infographic about some “strategies” for the game Rock-Paper-Scissors.
http://flowingdata.com/2010/07/30/how-to-win-rock-paper-scissors-every-time/
Although this graphic is a bit facetious, the author points out that there can be some real psychology behind playing the game.
I always thought it would be fun to build a simple computer simulation of the game, program different “players” with different strategies, and then run a bunch of tournaments and see what happens.
Appreciation Numbers
Prime Hunting
While at a hotel, waiting for some out-of-town friends to get ready, I went prime number hunting on the 11th floor.
I’m not especially good at factoring, so I just captured numbers that looked prime. I wasn’t thinking too hard about it, so I didn’t notice the one obvious non-prime until later, but the others looked pretty prime to me.
I expected more than one, but at least I didn’t come up empty.
Where are some other good places to go prime hunting?
Application Appreciation Challenge
Mathematics of Construction
One of the items on my to-do list was build something. To that end, I spent the afternoon turning a bunch of leftover lumber into a small table.
I’ve always admired those who can build elegant and functional things, but I came away from my project with an even greater appreciation for carpenters, woodworkers, builders, and the like. The measuring: how far are those legs spaced apart? The calculating: when all these pieces get put together, how tall is this thing supposed to be? The engineering: why are things rolling off my table? It’s a set of serious cerebral challenges on top of the obvious physical demands.
One theme that stood out as I slowly assembled my table was that of error propagation. A tiny error at an early stage (a millimeter or two of slant on a “flat” cut on a leg) can produce a sizable error in the end (things quickly rolling off your table).
It put me in mind of the classic differential problems in elementary calculus. For example, suppose you are measuring the diameter of sphere with a margin of error of +/- .1 mm: how much error might this generate in calculating the volume of the sphere? The surface area?
An even more complex problem is understanding how different errors interact with each other, which is something I experience frequently while putting this table together!