Jason Merrill’s Lawnmower Puzzle

Jason Merrill recently posted a fun geometry puzzle inspired by his work on the Lawnmower Math activity for Desmos.  Here’s my paraphrase of the puzzle:

Suppose a lawnmower is tethered to a circular peg in the middle of the lawn. As the lawnmower moves along its spiral path, the rope shortens as its winds around the peg. At the moment the lawnmower contacts the peg, how much rope remains uncoiled?

When I first considered this problem it seemed hard.  After some thought, it seemed obvious.  Then, after some more thought, it seemed hard again.  That’s the sign of a compelling problem!

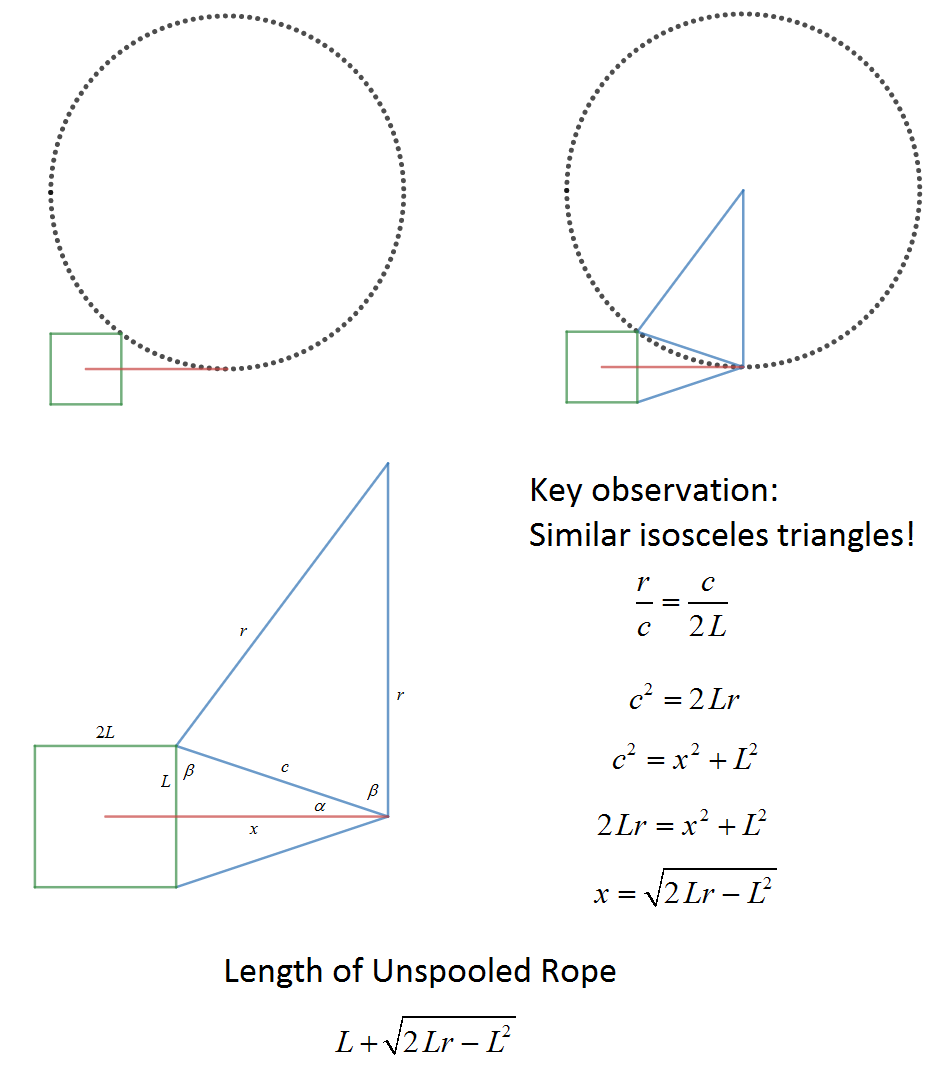
I enjoyed working out a solution, the heart of which I’ve included below.  Jason graciously included my solution in his post sharing his own, and he also does a wonderful job describing the journey of making simplifying assumptions, both mathematical and physical, that allow us to start moving toward a solution.  It’s the kind of work that often goes unmentioned in problem solving, especially in school mathematics, and this puzzle provides a nice opportunity to make that thinking transparent.

I highly recommend reading the puzzle and his solution at his blog.  Thanks for the fun problem, Jason!