Regents Recap — January 2016: No, It Wasn’t
“The graph below was created by an employee at a gas station.“
No, it wasn’t.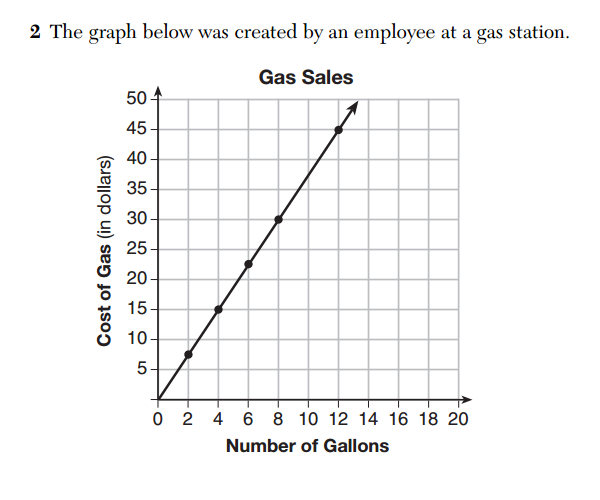
This problem from the January, 2016 Common Core Algebra Regents exam is just the latest in a long list of examples of absurdly contrived contexts on high-stakes exams. There seems to be a school of thought that believes we should go to great lengths to humanize test questions; I honestly can’t imagine why.
Not only does this fabricated context add nothing of value of this problem, it sends the message to students that applications of math are pointless and nonsensical. And as I argue in my talk g = 4, and Other Lies the Test Told Me, I fear that these messages add up over time.
Yet we know at least one good thing that came from this absurd test question: statistician Thomas Lumley was creatively inspired by this graph to imagine an amusing back-story! You can read it at his blog.
And you can find more of my critiques of New York State mathematics exams here.
Related Posts
- Regents Recaps
- Not Even Psuedo-Context
- Regents Recap — January 2016: No It Wasn’t
- g = 4, and Other Lies the Test Told Me
3 Comments
Jeanine · March 26, 2016 at 9:41 am
When my students and I come across questions like this, they make for a good laugh. We make conjectures about the person who came up with them, and what kind of life they lead. So maybe the intent is to get students to relax by injecting humor into the lesson. Right?
Jerome Dancis · March 26, 2016 at 9:47 am
The graph is mis-labelled.
The gas station’s “Cost of Gas” would be the money paid by the gas station to the gas distributer (wholesaler). But, “Gas Sales” would be the money paid by car owners (customers) to the gas station. These should be very different.
Also, if the station is selling two or three different hitest grades, then the graph of gas sales should be polygonal, not straight as various car drivers, buy the various grades of gas.
Yes, even if correct, these absurdly contrived contexts sends the message to students that applications of math are pointless and nonsensical.
russell · March 30, 2016 at 1:06 pm
re: NY State Geometry, specifically Engage NY curriculum, module 3, lesson 7. Alas, we can square a circle! The premise of the lesson is that the area of the base of a cone is equal to the area of the base of a pyramid with a quadrilateral base. There are many other problems with this particular curriculum. Another example is the rewriting of the vertex form of the equation of a parabola. What was once referred to as (1/4)p is now (1/2)p.