Regents Recap — June 2014: Which Graph is Steeper?
Here is another installment in my series reviewing the NY State Regents exams in mathematics.
The following question appeared on the June, 2014 Algebra 2 / Trig exam.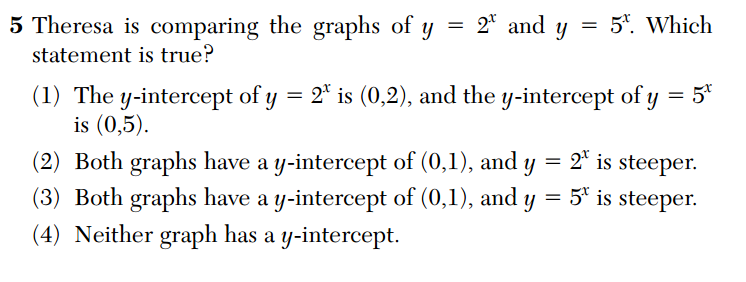 
To start, steeper is not a well-defined term, not in an Algebra 2 / Trig class, anyway. I’m not against using the word in everyday mathematics conversations, but I’m not a fan of putting it on an official exam like this. After all, I think these exams should model exemplary mathematical behavior. But that’s not the real issue here.
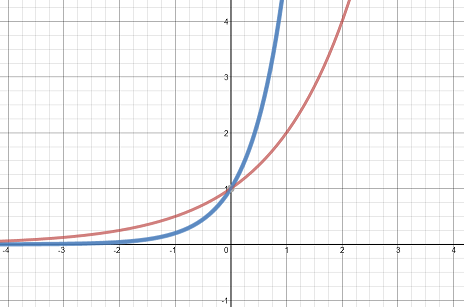
Even if we accept what steeper means, it can not be said that either graph is steeper than the other. Take a look: here, 
It seems pretty clear that the blue graph is steeper than the red on the right hand side, it also seems pretty clear that the red graph is steeper off to the left.
To be precise, the derivative of 
Thus, there really is no correct answer to this question. The answer key originally had (3) as the correct answer, but it is no truer than (2). Ultimately, a correction was issued for the problem, and both (2) and (3) were awarded full credit.
Mistakes are bound to happen when writing exams, and it’s good that a correction was ultimately issued. But this is a pretty obvious error. This question should not have made its way onto a high-stakes exam taken by tens of thousands of students. A thoughtful student might have been frustrated, confused, or disheartened confronting this question with no correct answer. Hopefully its impact didn’t extend beyond these two points.
3 Comments
Sendhil Revuluri · August 1, 2014 at 12:31 am
Fascinating example – thanks for pointing it out. These always surprise me, even having seen the “guts” of the item development, review, and revision process (and the many handoffs involved, with numerous opportunities for editing errors). In this case, would the ambiguity be resolved if the clause “at this point” were added at the end of options (2) and (3)?
MrHonner · August 1, 2014 at 7:23 am
I understand there are lots of opportunities for errors in this process, but it’s just hard to believe that not a single person saw this in development and thought to themselves, “Hmmmmm…..”.
The problem with specifying “at this point” is now you are specifically asking the student for the slope of the tangent line, which is not an Alg 2 / Trig topic.
pasmith · August 1, 2014 at 5:47 pm
Does mentioning Theresa make this question meaningfully different from “Which of the following statements about the graphs of y = 2^x and y = 5^x is true?”
To put the question another way: What box is being ticked by the statement that someone is comparing these graphs, but without any indication of their purpose for doing so?
If we were told why Theresa is interested in the steepness of these graphs then the question would be meaningfully different, since we might then be able to exclude the ambiguous region x < 0 as irrelevant to her purpose.