Regents Recap — January 2014: Fill-in-the-Blank Proofs
Here is another installment in my series reviewing the NY State Regents exams in mathematics.
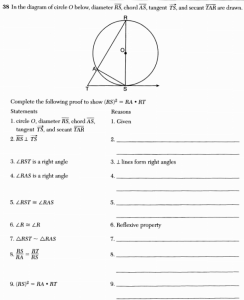
The January, 2014 Geometry exam included something I had not seen on a Regents exam: a fill-in-the-blank proof.
While I see some value in these kinds of problems in the teaching of two-column proofs, they shouldn’t be used on the final exam for a Geometry course. The goal of teaching proof is for students to develop the skills necessary to construct their own proofs from scratch. This problem reduces “proof” to a series of recall tasks.
So why not just ask the student to construct the proof from scratch? The rubric suggests the answer to that question.
While grading an open-ended proof is hard, checking off a list of six reasons is easy! Or so you would think.
Reports from colleagues who were grading this problem in a distributed grading center were disheartening. In particular, there was a lot of disagreement about what constituted appropriate justification in moving from
to
Apparently, teachers in the room wanted to accept “cross multiplying” as a legitimate reason, but would not accept “multiplication property of equality”. The site supervisor agreed, despite my colleagues’ objections.
Problems like this highlight the tendency to test what is easily tested and graded, not necessarily what’s important. And grading room stories like this should give pause to those who like to believe that these tests represent objective measures of learning or knowledge.

3 Comments
Jerome Dancis · February 26, 2014 at 11:30 am
“Cross-multiplication” is a non-understanding slogan, which has the numbers RA and RS climb up from the cellar and walk across the equal sign, as if it were a bridge.
“Cross-multiplication” is a procedure that some students will forget or garble over the summer. Worse, it trains students to violate what I have named “Epstein’s Rule”:
Epstein’s Rule. It is not all right to (or to teach students to) move numbers around in an equation.
“Subtracting 5 from both sides of an equation” uses the basic Rule: Equals minus equals are equals; instead of “moving the 5 to the other side” where it magically gets transformed to -5.
Violating Epstein’s Rule is an invitation and a common reason for creative mistakes.
The following example was circulated by Dr. Jerome Epstein[11] to a variety of classes.
Problem 3. Solve x/2 = 3x/4 – 1.
Many students (with standard instruction) learn how to do this problem, predictably many do not. Dr. Jerome Epstein observed that the most common creative error made was to incorrectly “cross-multiply” the two terms next to the equal sign while leaving the “1” alone, thereby obtaining the incorrect equation: 4x = 6x – 1.
Wendy Menard · February 26, 2014 at 8:53 pm
I believe I was in the room in which that debate took place (read http://hermathness.wordpress.com/2014/01/31/tricks-in-new-york/). My incredulity at the conversation was only superseded by my incredulity at the question on the exam. This is not the only evidence that the Geometry Regents tests what is easy to grade and to do, as the multiple questions on the equation of a circle suggests. I would love to see the exam truly assess the conceptual understanding of Geometry I try to bring my students to, but I’m not hopeful, especially after listening to some of the rationales that were given for accepting cross multiplication as a reason (‘that’s what they’ve been taught since they are in grade school’ was a favorite of mine).
CCSSIMath · February 26, 2014 at 10:11 pm
NY State Regents courses (and their grading) have long been anally retentive, such as distinguishing line segment “equality” vs. “congruence”, and then penalizing students for failure to “properly” notate = vs. ≅.
Such nitpicking, though, pales in comparison to the greater problem of putting into a high school course a proof that, even if done “from scratch”, at its most complex step requires nothing more than recognizing and stating that corresponding sides of similar triangles are proportional. The rest of the steps are elementary school math; lumping them all together does not make this high school caliber.
If high schools want to put/keep proofs in geometry, they should expose students to problems that develop high school level thinking, such as is necessary to tackle proofs like this:
https://docs.google.com/file/d/0B6lw97EHbvfHMTF2LXVycW51b3M/edit