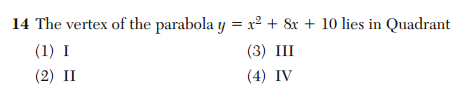Uncategorized

Teaching Testing Uncategorized

Regents Recap — January 2015: Admitting Mistakes

Here is another installment in my series reviewing the NY State Regents exams in mathematics.

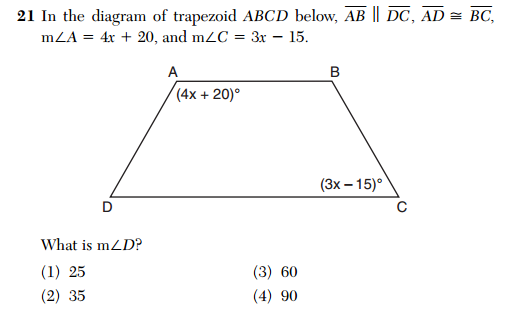
This is question 27 from the Geometry exam.

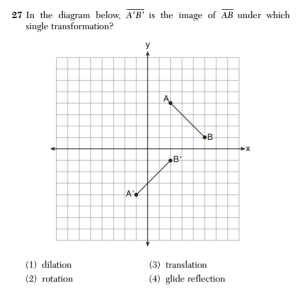This question has two correct answers: it is possible to map AB onto A’B’ using a glide reflection or a rotation. The original answer key indicated that (4) glide reflection was the correct answer. After the exam was administered, the state Department of Education issued a correction and told scorers to award full credit for both (2) and (4).

Mistakes happen, even on important exams that many people work hard to produce. But when mistakes are made, those responsible should accept responsibility, not equivocate.

Here’s the official correction from the state.

It’s hard to accept that the issue here was a lack of specificity in the wording of the question. The issue is that someone wrote a question without fully thinking through the mathematics, and then those tasked with checking the problem also failed to fully think through the mathematics. This isn’t a failure in communication; this is a failure in management and oversight.

And it has happened before. This example is particularly troubling, in which those responsible for producing these exams try to pretend that an egregious mathematical error is really just a lack of agreement about notation. Sometimes errors are just erased from the record with little or no explanation, and then, of course, there are the many mistakes that are never even acknowledged.

Mistakes are bound to happen. But by pretending that substantial errors are just misunderstandings, differences of opinion, or typos, the credibility of those responsible for these high-stakes exams suffers even further damage.

By MrHonner, 10 years10 years ago

Geometry Teaching Testing Uncategorized

Regents Recap — June 2014: When Good Math Becomes Bad Tests

Here is another installment in my series reviewing the NY State Regents exams in mathematics.

It is a true geometric wonder that a triangle’s medians always intersect at a single point. It is a remarkable and beautiful result, and the fact that the point of intersection is the centroid of the triangle makes it even more compelling.

This result should absolutely be a part of the standard Geometry curriculum. It important and beautiful mathematics, it extends a fundamental notion of mathematics (symmetry) in new ways, and it is readily accessible through folding, balancing, compass construction, and coordinate geometry.

But here’s what happens when high-stakes testing meets meaningful mathematics.

This wonderful result has been reduced to an easy-to-test trick: the centroid divides a median in a 2:1 ratio.

It’s not hard to see how such a fact can quickly become an instructional focus when it comes to centroids: if that’s how it’s going to be tested, that’s how it’s going to be taught. Of course, teachers should do more than just teach to a test, but there’s a lot riding on test results these days, and it’s hard to blame teachers for focusing on test scores when politicians, policy makers, and administrators tell them their jobs depend on it.

This is just one example of many, from one test and one state. This is an inseparable component of standardized testing, and it can be found in all content areas and at all levels. And for those who argue that the solution is simply to make better tests, keep this in mind: New York has been math Regents exams for over eighty years. Why haven’t we produced those better tests yet?

By MrHonner, 10 years10 years ago

Calculus Gave Me a Speeding Ticket

Years ago, one sunny Sunday afternoon, I was driving home from visiting friends at college and received a speeding ticket. I didn’t realize it at the time, but calculus played an important role in my citation.

You see, this was no ordinary speeding ticket, the kind where a police officer paces the offender or uses radar to measure a vehicle’s speed. My speed was calculated from an airplane high above the road. And the Mean Value Theorem clinched the case.

Aerial speed enforcement works like this: large marks painted on the road divide the highway into quarter-mile intervals. A pilot flying overhead uses a stopwatch to time a suspected speeder from one mark to the next. Say the pilot records a time of 12 seconds; a simple calculation converts one quarter mile per 12 seconds into 75 miles per hour; this information, the average speed on this interval, is radioed to the police on the ground who then stop and ticket the driver.

What I didn’t realize at the time was how crucial calculus is in all of this.

A fundamental theorem of calculus, the Mean Value Theorem (MVT), relates the average rate of change of a function with the instantaneous rate of change of the function. Suppose we have some function of time, $f(t)$ , and suppose that we know the value of this function at two times, say $f(t_1)$ and $f(t_2)$ . The average rate of change of $f(t)$ between $t_1$ and $t_2$ is

$f_{avg} = \frac{f(t_2) - f(t_1)}{t_2 - t_1}$

The MVT tells us that, as long as $f(t)$ is a differentiable function, then at some time between $t_1$ and $t_2$ , say at t = c, the instantaneous rate of change of $f(t)$ must have been equal to the average rate of change of $f(t)$ from $t_1$ and $t_2$ . That is,

$f'(c) = \frac{f(t_2) - f(t_1)}{t_2 - t_1}$

where $f'(x)$ is the derivative of $f(x)$ , the instantaneous rate of change of $f(x)$ .

What does this have to do with my speeding ticket? Well, as I’m moving along the highway in my car, the pilot records two values of my position function, $x(t)$ , at two different times, $t_1$ and $t_2$ . The pilot then computes my average speed

$x_{avg} = \frac{x(t_2) - x(t_1)}{t_2 - t_1}$

Here’s where calculus comes in. The Mean Value Theorem says that, at some point between those two times my instantaneous speed must have been equal to my average speed. If my average speed was above the legal limit, then at some time between $t_1$ and $t_2$ , my instantaneous speed must have been above the limit, and at that moment, I was guilty of speeding.

I wonder if it would have helped to argue that my position function wasn’t differentiable!

By MrHonner, 12 years12 years ago

Uncategorized

How Many Gregarious Mongolians Are There?

When I visited Mongolia 15 years ago, a young man approached us on the streets of Ulan Bator and offered to show us around. His name was Soyoloo, and we spent a few fun days hanging out with him.

I have many fond memories of Mongolia, so this NYT piece on Ulan Bator’s underground homeless population immediately caught my attention. The first picture is of a man named Soyoloo. I wondered if this was the same Soyoloo I met so long ago.

How likely is that? A naive estimate looks like this: half of the one million people in Ulan Bator are men, so there’s a 1 in 500,000 chance that a randomly-selected (that is, randomly photographed) man from Ulan Bator is the man I met 15 years ago. At 0.0002%, this does not seem very likely.

But here’s where conditional probability comes in. The man in the photograph isn’t just any randomly-selected man; he’s a randomly-selected man named Soyoloo. What I really want to know is, given the condition that a randomly selected man from Ulan Bator is named Soyoloo, what is the probability that he’s the man I met 15 years ago?

Naturally the answer depends on how many men in Ulan Bator are named Soyoloo. “Soyoloo” doesn’t appear on any list of most popular Mongolian names, so I am going to estimate that at most 0.5% of Mongolian men are named Soyoloo (this would be roughly equivalent to ‘Andrew’ in the US, the 35th most popular male name). This means that at most 2,500 men (0.5% of 500,000) in Ulan Bator are named Soyoloo. Therefore, if a man in Ulan Bator named Soyoloo is selected at random, there is a 1 in 2,500 chance (0.04%) it’s the man I met.

This is the power of conditional probability. The man in the photograph and the man I met share a common characteristic: being named Soyoloo. Knowing this condition greatly alters the probability that they are the same person.

In fact there’s another characteristic the two men share: they are both comfortable striking up relationships with foreign strangers, whether it’s me or a photojournalist. Let’s call this characteristic gregariousness. Now the question is, what is the probability that a randomly-selected gregarious male resident of Ulan Bator named Soyoloo is the person I met 15 years ago?

Well, how many gregarious Mongolians are there? Let’s say 5% of all Mongolians are gregarious. Then there are roughly 125 gregarious Mongolian men named Soyoloo in Ulan Bator, and thus, a 1 in 125 (0.8%) chance that the man in the photo is the same man I met. Not a lock, but not a longshot, either!

This probability may seem surprisingly high, given the distance in both time and space, but I have a feeling it’s him. His eyes look warmly familiar.

By MrHonner, 12 years12 years ago

Teaching Uncategorized

Regents Recap — January 2013: Question Design

Here is another installment in my series reviewing the NY State Regents exams in mathematics.

One consequence of scrutinizing standardized tests is a heightened sense of the role question design plays in constructing assessments.

Consider number 14 from the Integrated Algebra exam.

In order to correctly answer this question, the student has to do two things: they need to locate the vertex of a parabola; and they need to correctly name a quadrant.

Suppose a student gets this question wrong. Is it because they couldn’t find the vertex of a parabola, or because they couldn’t correctly name the quadrant? We don’t know.

Similarly, consider number 21 from the Geometry exam.

This is a textbook geometry problem, and there’s nothing inherently wrong with it. But if a student gets it wrong, we don’t know if they got it wrong because they didn’t understand the geometry of the situation, or because they couldn’t execute the necessary algebra.

Using student data to inform instruction is a big deal nowadays, and collecting student data is one of the justifications for the increasing emphasis on standardized exams. But is the data we’re collecting meaningful?

If a student gets the wrong answer, all we know is that they got the wrong answer. We don’t know why; we don’t know what misconceptions need to be corrected. In order to find out, we need to look at student work and intervene based on what we see.

And what if a student gets the right answer? Well, there is a non-zero chance they got it by guessing. In fact, on average, one out of four students who has no idea what the answer is will correctly guess the right answer. So a right answer doesn’t reliably mean that the student knows how to solve this problem, anyway.

So what then, exactly, is the purpose of these multiple choice questions?

By MrHonner, 12 years11 years ago

Uncategorized

Follow Mr Honner