MrHonner

3D Printing Geometry Teaching

Cavalieri’s Principle and 3D Printing

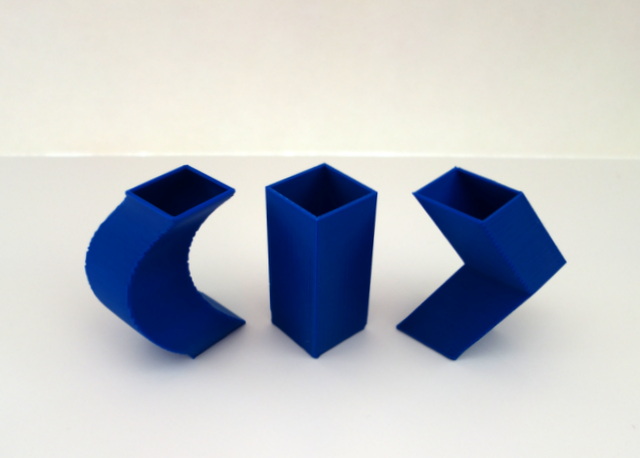
These 3D printed objects served as an excellent starting point for a classroom conversation on Cavalieri’s Principle.

Each shape above is an extrusion of the same square. In the middle, the square moves straight up; at left, the square travels in a complete circle from bottom to top; and at right, the square moves along a line segment and back.

The objects all have the same height. Since at every level they have the same cross-sectional area, by Cavalieri’s Principle they all have the same volume!

Cavalieri’s Principle is a simple but powerful idea, and one that can be easily demonstrated around the house. Here are some other examples using CDs and CD cases.

By MrHonner, 10 years10 years ago

Appreciation Numbers

05/21/2015 — Happy Derangement Day!

Today we celebrate a Derangement Day! Usually I call a day like today a permutation day because the digits of the day and month can be rearranged to form the year, but there’s something extra special about today’s date:

The numbers of the month and day are a derangement of the year: that is, they are a permutation of the digits of the year in which no digit remains in its original place!

Derangements pop up in some interesting places, and are connected to many rich mathematical ideas. The question “How many derangements of n objects are there?” is a fun and classic application of the principle of inclusion-exclusion. Derangements also figure in to some calculations of e and rook polynomials.

So enjoy Derangement Day! Today, it’s ok to be totally out of order.

By MrHonner, 10 years9 years ago

3D Printing Appreciation Geometry

3D-Printed Chmutov Surface

My most successful 3D print so far: a Chmutov surface!

Chmutov surfaces are algebraic surfaces that have interesting properties involving their singular points. This surface has equation

$2[x^2(3-4x^2)^2 + y^2(3-4y^2)^2 + z^2(3-4z^2)^2]=3$

And in addition to being quite beautiful, this Chmutov surface is already coming in handy!

By MrHonner, 10 years10 years ago

Appreciation Numbers

05/12/2015 — Happy Permutation Day!

Today we celebrate the third Permutation Day of the year! I call days like today permutation days because the digits of the day and the month can be rearranged to form the year.

Celebrate Permutation Day by mixing things up! Try doing things in a different order today. Just remember, for some operations, order definitely matters!

By MrHonner, 10 years10 years ago

Numbers Resources

Fun with SumBlox

We have been having fun with our SumBlox, which recently arrived. The number blocks are cleverly designed so that the height of each is proportional to its value.

Here we have the ten block

and here we have multiple mathematical ways to achieve the same height as the ten block: five + five, three + seven, and nine + one.

So far, playing with SumBlox seems like a fun way to build number sense and explore basic properties of addition like equivalence and commutativity. But there does seem to be one problem: I think they got the height of this block wrong!

By MrHonner, 10 years10 years ago

MrHonner

Follow Mr Honner